Sum Of The Infinite Geometric Series Calculator
Greels
Apr 05, 2025 · 5 min read
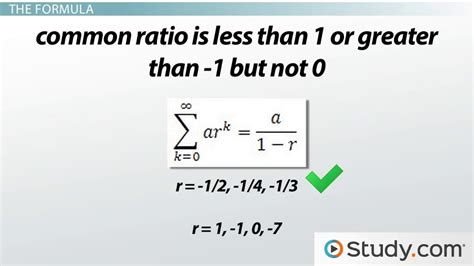
Table of Contents
Sum of the Infinite Geometric Series Calculator: A Comprehensive Guide
The concept of an infinite geometric series might seem daunting, but understanding its underlying principles and utilizing the right tools can make calculations straightforward. This article delves deep into the world of infinite geometric series, explaining the core concepts, providing practical examples, and introducing you to the power of a sum of the infinite geometric series calculator.
Understanding Infinite Geometric Series
A geometric series is a sequence where each term is found by multiplying the previous term by a constant value, called the common ratio (r). An infinite geometric series is simply a geometric series that continues indefinitely. The key to determining whether the sum of an infinite geometric series exists lies in the value of the common ratio.
Key Characteristics:
- Common Ratio (r): The constant value by which each term is multiplied.
- First Term (a): The initial value of the series.
- Convergence: An infinite geometric series converges (has a finite sum) only if the absolute value of the common ratio is less than 1: |r| < 1.
- Divergence: If |r| ≥ 1, the series diverges (its sum is infinite or undefined).
The Formula for the Sum of an Infinite Geometric Series
When an infinite geometric series converges (|r| < 1), its sum (S) can be calculated using the following formula:
S = a / (1 - r)
Where:
- S represents the sum of the infinite geometric series.
- a represents the first term of the series.
- r represents the common ratio.
This formula provides a concise and elegant way to determine the sum, eliminating the need for tedious calculations involving an infinite number of terms.
When to Use an Infinite Geometric Series Calculator
While the formula is relatively simple, manually calculating the sum can be prone to errors, especially when dealing with complex or lengthy series. This is where an infinite geometric series calculator becomes an invaluable tool. These calculators automate the calculation, ensuring accuracy and efficiency. They are particularly helpful in:
- Complex Calculations: When the first term or common ratio involves fractions, decimals, or radicals, the calculator streamlines the process.
- Time Constraints: Quickly obtaining the sum is crucial in many applications, and a calculator significantly reduces calculation time.
- Minimizing Errors: Human error is inevitable during manual calculations, especially with larger numbers or more complicated expressions. Calculators drastically reduce the risk of errors.
- Educational Purposes: Calculators can be used as learning aids to verify manual calculations and build a stronger understanding of the concepts.
Utilizing an Infinite Geometric Series Calculator: A Step-by-Step Guide
While the specific interface of calculators may vary, the basic steps remain consistent:
- Identify the First Term (a): Determine the initial value of the geometric series.
- Identify the Common Ratio (r): Calculate the constant value by which each term is multiplied to obtain the next. This is done by dividing any term by its preceding term. Ensure the ratio is consistent throughout the series.
- Verify Convergence: Check that |r| < 1. If this condition is not met, the series diverges, and the calculator (or the formula) will not provide a meaningful sum.
- Input Values: Enter the values of 'a' and 'r' into the respective fields of the calculator.
- Calculate: Click the "Calculate" or equivalent button. The calculator will compute the sum (S) based on the formula.
- Interpret Results: Carefully examine the result provided by the calculator. Make sure the answer makes sense in the context of the problem.
Practical Applications of Infinite Geometric Series
Infinite geometric series appear in diverse fields, showcasing their practical relevance beyond theoretical mathematics:
1. Physics
- Bouncing Ball: Imagine a ball dropped from a height 'h'. It bounces back to a fraction 'r' of its previous height. The total distance traveled by the ball is an infinite geometric series, useful for modeling real-world scenarios involving energy loss.
2. Economics
- Compound Interest: Calculating the future value of an investment with continuous compounding (theoretically, infinitely many compounding periods) involves an infinite geometric series.
3. Computer Science
- Fractals: The intricate patterns of fractals, like the Mandelbrot set, are generated using iterative processes often modeled by infinite geometric series. Understanding these series helps in understanding and creating these complex designs.
4. Engineering
- Signal Processing: Analyzing signals with decaying amplitudes, such as damped oscillations, often requires summing infinite geometric series.
5. Medicine
- Drug Dosage: Certain medication dosage models, where the drug is metabolized and then re-administered, can be approximated using infinite geometric series. The steady-state concentration of the drug in the body can be determined using this concept.
Advanced Concepts and Considerations
While the basic formula is straightforward, some scenarios necessitate a deeper understanding:
1. Series with Negative Common Ratio
When the common ratio is negative, the terms of the series alternate between positive and negative values. The formula still applies, but the result reflects this alternating behavior.
2. Dealing with Complex Numbers
The formula for the sum of an infinite geometric series also extends to series with complex numbers as the first term or common ratio. However, understanding complex number arithmetic becomes crucial in these calculations.
3. Approximations
In some real-world applications, the exact sum of an infinite series might not be necessary. Using partial sums (summing a finite number of terms) provides a reasonable approximation, especially when the common ratio is small.
Beyond the Calculator: Strengthening Your Understanding
While calculators offer efficiency, a strong foundational understanding of geometric series remains vital. Practicing manual calculations with simpler series helps solidify your grasp of the underlying concepts. Furthermore, exploring the convergence and divergence aspects thoroughly enhances your analytical skills.
Conclusion: Embracing the Power of Calculation and Understanding
The sum of the infinite geometric series calculator is an indispensable tool for accurately and efficiently calculating sums. However, its optimal use is coupled with a robust understanding of the fundamental principles and limitations of infinite geometric series. Mastering both the theoretical underpinnings and the practical application of calculators ensures proficiency in handling this essential mathematical concept across numerous disciplines. By combining the speed and precision of calculators with a thorough understanding of the mathematical theory, you can tackle complex problems with confidence and accuracy. Remember to always check your results to ensure they make sense within the context of the problem.
Latest Posts
Latest Posts
-
How Many Inches In 61 Cm
Apr 05, 2025
-
How Many Miles Is 38 Km
Apr 05, 2025
-
What Is 80 Percent Of 18
Apr 05, 2025
-
1 7 3 3 7n 2 7
Apr 05, 2025
-
What Is 5 Percent Of 2500
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Sum Of The Infinite Geometric Series Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
