Derivative Of Sqrt 1 X 2
Greels
Apr 03, 2025 · 5 min read
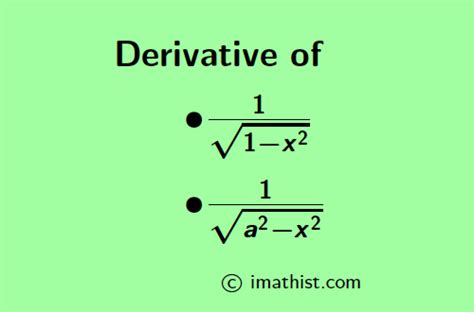
Table of Contents
Decoding the Derivative of √(1 + x²) : A Comprehensive Guide
The derivative of √(1 + x²), a seemingly simple expression, unveils a fascinating journey into the world of calculus. This seemingly straightforward function holds significant importance in various fields, from physics and engineering to computer graphics and machine learning. Understanding its derivative unlocks the door to solving complex problems related to curves, slopes, and optimization. This article will meticulously explore the derivation, its applications, and related concepts, offering a comprehensive understanding for students and enthusiasts alike.
Understanding the Function: √(1 + x²)
Before diving into the derivative, let's understand the function itself. √(1 + x²) represents the square root of the sum of one and the square of x. Graphically, this function describes the upper half of a hyperbola. This hyperbola plays a crucial role in various mathematical models and physical phenomena. For instance, it's intimately linked to the calculation of arc lengths and the representation of certain curves in space.
Applying the Chain Rule: The Key to Differentiation
Calculating the derivative of √(1 + x²) requires a crucial technique: the chain rule. The chain rule allows us to differentiate composite functions—functions within functions. In this case, our composite function is the square root function acting on (1 + x²).
The chain rule states that the derivative of a composite function f(g(x)) is given by:
d/dx [f(g(x))] = f'(g(x)) * g'(x)
Here's how we apply the chain rule to our function:
-
Identify the inner and outer functions:
- Outer function: f(u) = √u (where u = 1 + x²)
- Inner function: g(x) = 1 + x²
-
Find the derivatives of the inner and outer functions:
- Derivative of the outer function: f'(u) = 1/(2√u)
- Derivative of the inner function: g'(x) = 2x
-
Apply the chain rule:
d/dx [√(1 + x²)] = f'(g(x)) * g'(x) = [1/(2√(1 + x²))] * (2x)
-
Simplify the result:
d/dx [√(1 + x²)] = x/√(1 + x²)
Therefore, the derivative of √(1 + x²) is x/√(1 + x²).
Visualizing the Derivative: Slope and Tangent Lines
The derivative, x/√(1 + x²), represents the instantaneous rate of change of the function √(1 + x²) at any given point x. Geometrically, it represents the slope of the tangent line to the curve of √(1 + x²) at that point. The slope is positive for positive x values and negative for negative x values, reflecting the increasing and decreasing nature of the function.
Consider the point (1, √2) on the curve. At this point, the derivative is 1/√2, indicating the tangent line has a slope of 1/√2. This visual representation provides a deeper intuitive understanding of the derivative's meaning.
Applications of the Derivative: Beyond the Textbook
The derivative of √(1 + x²) has far-reaching applications in various fields:
1. Physics and Engineering:
- Calculating Arc Length: The derivative is crucial in calculating the arc length of the hyperbola represented by √(1 + x²). Arc length calculations are essential in many engineering applications, such as designing curved structures or calculating the distance traveled along a curved path.
- Velocity and Acceleration: In physics, if √(1 + x²) represents the displacement of an object as a function of time, the derivative gives its velocity, and the second derivative gives its acceleration.
- Optimization Problems: Finding maxima and minima of functions involving √(1 + x²) often involves solving equations where the derivative is set to zero. This has applications in optimizing designs, minimizing energy consumption, and maximizing efficiency.
2. Computer Graphics and Image Processing:
- Curve Representation: The function √(1 + x²) can be used to represent curves in computer graphics. The derivative helps in calculating tangent vectors and normals to these curves, which is essential for rendering and shading techniques.
- Image Transformations: Image transformations, such as rotations and scaling, often involve mathematical functions like √(1 + x²). The derivative plays a crucial role in understanding how these transformations affect the image.
3. Machine Learning and Data Science:
- Gradient Descent: In machine learning algorithms, such as gradient descent, the derivative is used to find the direction of steepest descent in optimizing model parameters. Functions related to √(1 + x²) might appear within loss functions, and their derivatives guide the optimization process.
- Mathematical Modeling: This function and its derivative could be incorporated into complex models related to various phenomena, requiring derivative calculations for analysis and optimization.
Advanced Concepts and Extensions
The understanding of the derivative of √(1 + x²) opens doors to more advanced concepts:
1. Implicit Differentiation:
If √(1 + x²) is part of a more complex equation (e.g., x² + y² = 1), implicit differentiation would be used to find dy/dx. This involves differentiating both sides of the equation with respect to x, treating y as a function of x, and then solving for dy/dx.
2. Higher-Order Derivatives:
The second derivative of √(1 + x²) can be found by differentiating the first derivative (x/√(1 + x²)). This represents the rate of change of the slope, offering insights into the curvature of the original function.
3. Integration:
The integral of x/√(1 + x²) is related to the original function √(1 + x²). This involves techniques like substitution to solve the integral and is directly connected to finding areas under the curve.
Conclusion: Mastering the Derivative's Power
The seemingly simple derivative of √(1 + x²) unveils a rich tapestry of mathematical concepts and practical applications. From understanding the slope of tangent lines to solving intricate problems in physics, engineering, and computer science, mastering this derivative enhances one's ability to analyze and model various phenomena. This comprehensive guide provides a solid foundation for further exploration of calculus and its role in our understanding of the world around us. By understanding its derivation, applications, and related concepts, you've unlocked a powerful tool within the realm of mathematics and its various fields of application. This knowledge allows you to approach more complex problems with confidence and precision, expanding your skillset and broadening your understanding of the interconnectedness of mathematical principles.
Latest Posts
Latest Posts
-
What Is 1 83 M In Feet
Apr 03, 2025
-
Cuanto Es 79 Kg En Libras
Apr 03, 2025
-
How Many Ounces Is 230 Grams
Apr 03, 2025
-
How Many Inches Is 44 Feet
Apr 03, 2025
-
Fundamental Theorem Of Calculus Part 2 Calculator
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Derivative Of Sqrt 1 X 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
