Fundamental Theorem Of Calculus Part 2 Calculator
Greels
Apr 03, 2025 · 5 min read
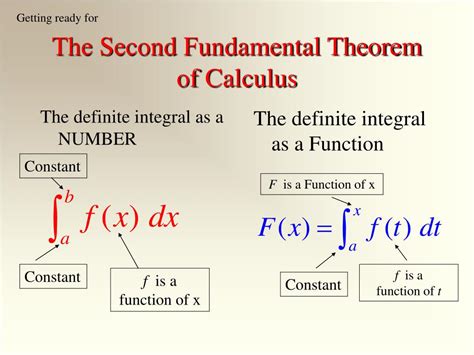
Table of Contents
Fundamental Theorem of Calculus Part 2 Calculator: A Comprehensive Guide
The Fundamental Theorem of Calculus, Part 2, is a cornerstone of calculus, providing a powerful tool for evaluating definite integrals. Understanding this theorem is crucial for anyone studying calculus, and having a grasp of its applications is vital for solving various problems in science, engineering, and mathematics. This article will delve deep into the Fundamental Theorem of Calculus Part 2, explain its intricacies, and explore how calculators can be utilized to enhance its application. We'll also touch upon the practical limitations and considerations when employing calculator assistance.
Understanding the Fundamental Theorem of Calculus Part 2
The Fundamental Theorem of Calculus Part 2 states that if f is continuous on the interval [a, b] and F is an antiderivative of f on [a, b], then:
∫<sub>a</sub><sup>b</sup> f(x) dx = F(b) - F(a)
This theorem elegantly connects differentiation and integration, demonstrating that integration is essentially the reverse process of differentiation. Instead of directly evaluating a definite integral using Riemann sums (a tedious process, especially for complex functions), we can find an antiderivative and evaluate it at the limits of integration. This significantly simplifies the calculation of definite integrals.
Key Concepts and Terminology
Before we delve into calculator applications, let's reinforce some crucial concepts:
- Definite Integral: Represents the signed area between a curve and the x-axis over a specific interval [a, b].
- Antiderivative (or Indefinite Integral): A function whose derivative is the original function. It's denoted as ∫ f(x) dx and includes an arbitrary constant of integration (C).
- Limits of Integration: The values a and b defining the interval over which the integral is calculated.
- Continuous Function: A function without any abrupt jumps or breaks within a given interval. Continuity is a prerequisite for applying the Fundamental Theorem of Calculus Part 2.
Utilizing Calculators for Evaluating Definite Integrals
While the Fundamental Theorem of Calculus Part 2 provides the theoretical framework, calculators serve as invaluable tools for tackling the practical aspects of evaluating definite integrals, especially those involving complex functions. Many calculators, from basic scientific calculators to sophisticated graphing calculators and computer algebra systems (CAS), possess built-in functionalities for calculating definite integrals.
Different Calculator Types and Their Capabilities
The capabilities of calculators in handling definite integrals vary significantly depending on their type:
-
Basic Scientific Calculators: These calculators typically handle simpler functions and often require manual input of the antiderivative. They lack symbolic integration capabilities. You would need to find the antiderivative yourself, then substitute the limits of integration.
-
Graphing Calculators (e.g., TI-84, TI-Nspire): These calculators offer more advanced functionalities, often including numerical integration routines. They can approximate the definite integral without requiring you to find the antiderivative explicitly. This is particularly useful for functions that lack elementary antiderivatives.
-
Computer Algebra Systems (CAS) (e.g., Mathematica, Maple, Wolfram Alpha): These powerful software packages provide symbolic integration capabilities. They can find the exact antiderivative of a wide range of functions and then evaluate it at the limits of integration to provide the exact value of the definite integral (when possible).
Step-by-Step Guide to Using a Calculator
The specific steps for calculating a definite integral using a calculator will vary depending on the calculator model. However, the general approach is similar:
-
Input the Function: Enter the function f(x) accurately. Pay close attention to parentheses and correct syntax.
-
Specify the Limits of Integration: Enter the lower limit (a) and the upper limit (b) of the integration interval.
-
Execute the Integration Command: Most calculators have a dedicated integration function, often denoted by ∫ or a similar symbol. Use this function to initiate the calculation.
-
Interpret the Result: The calculator will display the result, which is the value of the definite integral. Remember that the result might be an approximation (especially for numerical methods) rather than the exact value.
Example using a hypothetical calculator syntax:
Let's evaluate ∫<sub>1</sub><sup>3</sup> x² dx. A typical calculator syntax might look like this:
∫(x², x, 1, 3)
The calculator should return the result: 26/3 or approximately 8.667.
Practical Limitations and Considerations
While calculators are extremely helpful, it's essential to understand their limitations:
-
Approximation Errors: Numerical integration methods used by some calculators introduce approximation errors. The accuracy of the result depends on the method used and the complexity of the function.
-
Inability to Handle Certain Functions: Some calculators might struggle with functions that lack elementary antiderivatives or those with singularities (points where the function is undefined).
-
Understanding the Underlying Math: Relying solely on calculators without understanding the Fundamental Theorem of Calculus Part 2 can hinder the development of crucial mathematical skills. Calculators should be used as tools to enhance understanding, not replace it.
-
Correct Input: Incorrect function input or limits of integration will lead to inaccurate results. Always double-check your input before executing the calculation.
Advanced Applications and Extensions
The Fundamental Theorem of Calculus Part 2 has widespread applications beyond basic integration problems. Here are a few examples:
-
Calculating Areas and Volumes: Definite integrals are used extensively in geometry to calculate areas under curves, areas between curves, and volumes of solids of revolution.
-
Physics and Engineering: Numerous applications in physics and engineering involve calculating work, displacement, and other quantities using definite integrals.
-
Probability and Statistics: Definite integrals are crucial in probability theory for calculating probabilities and expected values.
-
Economics and Finance: Definite integrals are used in economic modeling for concepts such as consumer surplus and producer surplus.
Conclusion
The Fundamental Theorem of Calculus Part 2 is a fundamental concept in calculus with far-reaching applications. Calculators significantly aid in evaluating definite integrals, especially for complex functions. However, it's imperative to understand the underlying mathematical principles and the limitations of calculator-based approaches. By combining a solid understanding of the theorem with the computational power of calculators, you can efficiently and effectively solve a wide range of problems involving definite integration. Remember, the calculator is a tool to assist you; it shouldn't replace your understanding of the underlying mathematics. Always strive for a balanced approach, combining theoretical knowledge with practical application.
Latest Posts
Latest Posts
-
How Many Inches Is 127 Cm
Apr 04, 2025
-
What Day Will It Be In 37 Days
Apr 04, 2025
-
62 Inches Is How Many Centimeters
Apr 04, 2025
-
1600 Meters Is How Many Feet
Apr 04, 2025
-
How Much Is 16kg In Pounds
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Fundamental Theorem Of Calculus Part 2 Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
