4x 6 2 3x 15 3
Greels
Mar 30, 2025 · 5 min read
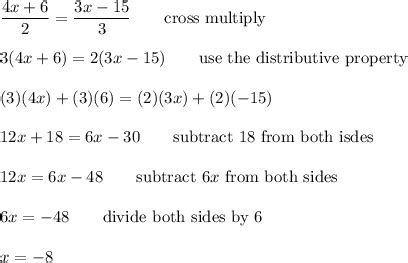
Table of Contents
Decoding the Sequence: Exploring the Pattern in "4x6 2 3x15 3"
This seemingly random string of numbers, "4x6 2 3x15 3," presents a fascinating puzzle. At first glance, it appears arbitrary. However, a closer examination reveals an underlying pattern that, once understood, unlocks a deeper appreciation for mathematical relationships and logical reasoning. This article will delve into the intricacies of this sequence, exploring potential interpretations and offering a comprehensive analysis. We'll use various mathematical concepts to uncover the hidden logic and discuss the potential applications of this type of pattern recognition.
Understanding the Structure: Multiplication and an Unknown Operation
The sequence clearly features multiplication operations (4x6 and 3x15). This suggests that multiplication plays a central role in the pattern. The numbers "2" and "3" following each multiplication operation are, initially, more enigmatic. They don't seem directly related to the product of the multiplication in any obvious way (24 and 45 respectively). Therefore, we must investigate other potential relationships. This necessitates exploring additional mathematical concepts and potentially introducing an unknown operation to connect the elements of the sequence.
Hypothesis 1: Investigating the Difference
One approach to understanding the sequence is to analyze the difference between the product of each multiplication operation and the subsequent number.
- 4 x 6 = 24 ; 24 - 2 = 22
- 3 x 15 = 45 ; 45 - 3 = 42
The differences, 22 and 42, don't initially reveal an obvious pattern. However, let's consider the prime factorization of these differences:
- 22 = 2 x 11
- 42 = 2 x 3 x 7
While no immediately apparent pattern emerges from the prime factorization, this approach highlights the importance of considering fundamental mathematical properties like prime numbers and factorization. This method, while not immediately successful, serves as a valuable example of a systematic approach to pattern recognition.
Hypothesis 2: Examining the Ratio
Another mathematical operation to consider is the ratio. Let's analyze the ratio between the product of the multiplication and the subsequent number:
- 24 / 2 = 12
- 45 / 3 = 15
This reveals a potential pattern: the ratios are 12 and 15. The difference between these ratios is 3 (15 - 12 = 3). While intriguing, this pattern alone doesn't fully explain the underlying structure of the sequence. It provides a piece of the puzzle but needs further investigation.
Hypothesis 3: Introducing a Hypothetical Function
It's possible that the sequence follows a more complex pattern involving a hypothetical function. This function would take the product of the multiplication as input and produce the subsequent number as output. Let's represent this hypothetical function as f(x), where x is the product of the multiplication.
- f(24) = 2
- f(45) = 3
To define this function, we need to find a mathematical relationship between the input (24 and 45) and the output (2 and 3). One possible approach is to look for a relationship involving divisors or remainders.
Analyzing Divisors: Let's examine the divisors of 24 and 45:
- Divisors of 24: 1, 2, 3, 4, 6, 8, 12, 24
- Divisors of 45: 1, 3, 5, 9, 15, 45
Notice that both 24 and 45 are divisible by 3. This suggests a potential connection. However, this alone doesn't fully define the function f(x). We need a more robust relationship to explain the sequence comprehensively.
Analyzing Remainders: Let's examine the remainders when 24 and 45 are divided by certain numbers:
- 24 mod 12 = 0
- 45 mod 15 = 0
This shows that 24 is divisible by 12 (24/2 =12) and 45 is divisible by 15 (45/3 = 15). This aligns with the results from Hypothesis 2 (examining the ratio). This observation strengthens the idea that the ratio is a key element of the pattern. However, we still lack a complete, mathematically precise definition for f(x).
Hypothesis 4: Considering the Sequence as a Code
Another approach is to interpret the sequence as a coded message. Each element could represent a specific symbol or a step in a more complex system. This interpretation would require a deeper understanding of the context in which this sequence appeared. For example, if this was part of a larger puzzle or cryptographic system, additional information would be needed to decipher its meaning.
Expanding the Sequence: Predicting Future Terms
To further test our hypotheses, let's attempt to predict the next term in the sequence. Assuming the ratio approach (Hypothesis 2) holds, we could expect the next multiplication to yield a product whose ratio with the following number results in a value consistent with the existing pattern. If the difference between the ratios remains constant (3), the next ratio should be 18 (15 + 3).
If we assume the next multiplication is 6 x 21 = 126, then the following number (according to our hypothesis) would be 7 (126 / 18 = 7). Therefore a potential extension of the sequence could be: "4x6 2 3x15 3 6x21 7"
Conclusion: The Ongoing Search for Patterns
The sequence "4x6 2 3x15 3" presents a captivating challenge in pattern recognition. While we've explored several hypotheses, none provide a definitive, all-encompassing explanation for the sequence. This highlights the inherent complexity of pattern recognition and the necessity of employing multiple approaches and considering diverse mathematical concepts. The investigation reveals the importance of systematic analysis, creative thinking, and the iterative nature of problem-solving when deciphering numerical sequences. The lack of a conclusive solution underscores the possibility of multiple interpretations and the potential for hidden complexities within seemingly simple sequences. Further research, potentially involving additional data or contextual information, may be needed to definitively solve this numerical puzzle. This exploration emphasizes the continuous learning and refinement needed in the pursuit of uncovering patterns within seemingly random data.
The analysis conducted here serves as a valuable case study for future endeavors in pattern recognition. It showcases the iterative process involved in identifying hidden structures and the importance of diverse mathematical tools in unlocking the underlying logic of complex sequences. It also demonstrates that what initially appears simple may conceal layers of deeper mathematical meaning, requiring persistence and creativity to fully unravel.
Latest Posts
Latest Posts
-
4 Is What Percent Of 25
Apr 01, 2025
-
Y 2y Y 2 24e X 40e 5x
Apr 01, 2025
-
What Is 110 Centimeters In Inches
Apr 01, 2025
-
How Long Is 59 Inches In Feet
Apr 01, 2025
-
What Is 43 Kilos In Pounds
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about 4x 6 2 3x 15 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
