Write The Polynomial In Standard Form.
Greels
Mar 27, 2025 · 5 min read
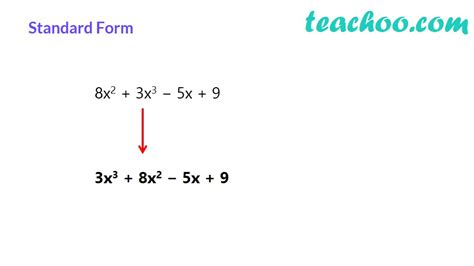
Table of Contents
Writing Polynomials in Standard Form: A Comprehensive Guide
Polynomials are fundamental building blocks in algebra, appearing in countless mathematical applications. Understanding how to write a polynomial in standard form is crucial for various algebraic manipulations, simplifying expressions, and solving equations. This comprehensive guide will delve into the intricacies of writing polynomials in standard form, covering definitions, examples, and advanced applications.
What is a Polynomial?
Before diving into standard form, let's solidify our understanding of polynomials themselves. A polynomial is an expression consisting of variables (often represented by x, y, etc.) and coefficients, combined using addition, subtraction, and multiplication, but never division by a variable. Each term in a polynomial consists of a coefficient and a variable raised to a non-negative integer power.
Examples of Polynomials:
- 3x² + 5x - 7
- 2y⁴ - 6y² + 1
- 4x³y² + 2xy - 9
- 5 (this is a constant polynomial)
Examples of Expressions that are NOT Polynomials:
- 1/x + 2 (division by a variable)
- √x + 5 (variable raised to a non-integer power)
- x⁻² + 3x (negative exponent)
Understanding the Degree of a Polynomial
The degree of a polynomial is determined by the highest power of the variable present in the expression. This is crucial when arranging a polynomial into standard form.
Examples:
- 3x² + 5x - 7: The degree is 2 (highest power of x).
- 2y⁴ - 6y² + 1: The degree is 4 (highest power of y).
- 4x³y² + 2xy - 9: This is a polynomial in two variables. The degree is 5 (3 + 2, the sum of the highest powers of x and y).
- 5: The degree is 0 (a constant polynomial).
Standard Form of a Polynomial
The standard form of a polynomial arranges the terms in descending order of their degrees. This means that the term with the highest power of the variable appears first, followed by the term with the next highest power, and so on, until the constant term (if any).
Steps to Write a Polynomial in Standard Form:
- Identify the terms: Break down the polynomial into its individual terms.
- Determine the degree of each term: Find the exponent of the variable in each term.
- Arrange the terms: Order the terms in descending order based on their degrees.
- Combine like terms (if necessary): If the polynomial contains like terms (terms with the same variable and exponent), combine them by adding or subtracting their coefficients.
Examples:
-
Write 5x - 7 + 3x² in standard form:
The terms are 5x, -7, and 3x². Their degrees are 1, 0, and 2 respectively. In standard form, this polynomial becomes 3x² + 5x - 7.
-
Write 2y + 4y³ - 6y² + 1 in standard form:
The terms are 2y, 4y³, -6y², and 1. Their degrees are 1, 3, 2, and 0 respectively. The standard form is 4y³ - 6y² + 2y + 1.
-
Write 2x²y³ - 5xy⁴ + 3x³y + 1 in standard form:
This is a polynomial in two variables. The degrees of the terms are 5, 5, 4, and 0. Arranging in descending order considering the sum of the powers: 2x²y³ - 5xy⁴ + 3x³y + 1 becomes 3x³y - 5xy⁴ + 2x²y³ + 1
Working with Polynomials in Standard Form: Applications
Writing polynomials in standard form is not merely an exercise in organization. It's a fundamental step in various algebraic operations:
1. Polynomial Addition and Subtraction:
Adding or subtracting polynomials is simplified significantly when they are in standard form. Like terms are easily identified and combined.
Example:
Add (3x² + 5x - 7) and (x² - 2x + 4).
Both polynomials are already in standard form. Adding them:
(3x² + 5x - 7) + (x² - 2x + 4) = (3 + 1)x² + (5 - 2)x + (-7 + 4) = 4x² + 3x - 3
2. Polynomial Multiplication:
While multiplication doesn't inherently require standard form, the resulting polynomial is often easier to simplify and express in standard form afterwards.
Example:
Multiply (2x + 3) and (x² - x + 1).
(2x + 3)(x² - x + 1) = 2x(x² - x + 1) + 3(x² - x + 1) = 2x³ - 2x² + 2x + 3x² - 3x + 3 = 2x³ + x² - x + 3.
The result is in standard form.
3. Polynomial Division:
Polynomial long division and synthetic division are greatly facilitated by having the polynomials in standard form. Missing terms (terms with a coefficient of 0) are often represented as placeholders to maintain proper alignment during the division process.
4. Finding Roots (Zeros) of Polynomials:
The standard form provides a clear picture of the highest power of the polynomial, indicating the maximum number of roots the polynomial can have. Various methods for finding roots, such as factoring or the quadratic formula (for degree 2 polynomials), are more easily applied when the polynomial is in standard form.
5. Graphing Polynomials:
The standard form is helpful in determining the end behavior of a polynomial function (what happens to the y-values as x approaches positive or negative infinity). The leading term (the term with the highest degree) dictates the overall shape of the graph for large values of x.
Advanced Concepts and Challenges:
-
Polynomials in Multiple Variables: The principle of arranging terms in descending order of degree extends to polynomials with multiple variables. A consistent ordering scheme (e.g., lexicographical order) should be applied.
-
Dealing with Missing Terms: When a polynomial lacks a term with a particular degree, it's essential to include a term with a coefficient of zero as a placeholder when writing the polynomial in standard form to maintain proper alignment during operations like addition, subtraction or division. For example, x³ + 1 would be written as x³ + 0x² + 0x + 1 in standard form to clearly show the absence of the x² and x terms.
-
Complex Coefficients: Polynomials can have complex numbers (numbers involving the imaginary unit i) as coefficients. The process of writing them in standard form remains the same, albeit with the added complexity of handling complex numbers.
Conclusion:
Writing polynomials in standard form is a fundamental skill in algebra with far-reaching implications. It streamlines various algebraic operations, aids in understanding the properties of polynomials, and simplifies tasks like solving equations and graphing functions. Mastering this skill is crucial for progressing to more advanced algebraic concepts. By consistently practicing writing polynomials in standard form and applying these techniques to various problems, a strong foundation in algebra will be built. The ability to confidently manipulate polynomials in their standard form is essential for success in higher-level mathematics and its applications in science and engineering.
Latest Posts
Latest Posts
-
How Long Is 90 Inches In Feet
Mar 30, 2025
-
How Many Feet Is 101 In
Mar 30, 2025
-
How Many Inches Is 29 Mm
Mar 30, 2025
-
How Many Kg Is 152 Pounds
Mar 30, 2025
-
5000 Km Is How Many Miles
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about Write The Polynomial In Standard Form. . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
