What Is The Integral Of Xlnx
Greels
Apr 05, 2025 · 4 min read
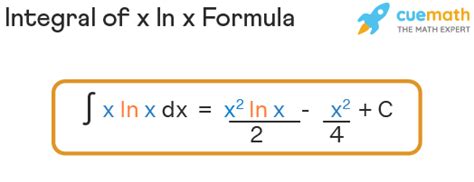
Table of Contents
What is the Integral of xlnx? A Comprehensive Guide
The integral of xlnx is a classic example in calculus that showcases the power of integration by parts. While seemingly straightforward, understanding its solution requires a solid grasp of logarithmic properties and integration techniques. This comprehensive guide will delve into the step-by-step process of solving this integral, explore its applications, and touch upon related integration problems.
Understanding Integration by Parts
Before tackling the integral of xlnx, let's refresh our understanding of integration by parts. This technique is crucial for solving integrals involving the product of two functions. The formula for integration by parts is derived from the product rule of differentiation and is expressed as:
∫u dv = uv - ∫v du
Where:
uandvare functions of x.duanddvrepresent their respective differentials.
Choosing the appropriate u and dv is key to successfully applying this method. A helpful mnemonic device is LIATE, which prioritizes the order of function types:
- Logarithmic functions
- Inverse trigonometric functions
- Algebraic functions
- Trigonometric functions
- Exponential functions
This order suggests that logarithmic functions should ideally be chosen as u when present in the integrand.
Solving the Integral of xlnx
Now, let's apply integration by parts to solve ∫xlnx dx.
-
Choose u and dv:
Following the LIATE rule, we select:
u = lnx=>du = (1/x) dxdv = x dx=>v = (1/2)x²
-
Apply the Integration by Parts Formula:
Substituting our choices into the formula, we get:
∫xlnx dx = (1/2)x²lnx - ∫(1/2)x² * (1/x) dx
-
Simplify and Integrate:
Simplifying the remaining integral, we have:
∫xlnx dx = (1/2)x²lnx - (1/2)∫x dx
Now, integrate the simplified expression:
∫xlnx dx = (1/2)x²lnx - (1/2) * (1/2)x² + C
-
Final Solution:
Combining the terms and adding the constant of integration (C), we arrive at the final solution:
∫xlnx dx = (1/2)x²lnx - (1/4)x² + C
Verification Through Differentiation
To verify our solution, we can differentiate the result using the product rule and chain rule:
d/dx [(1/2)x²lnx - (1/4)x² + C] = (1/2)[2xlnx + x²(1/x)] - (1/2)x = xlnx + (1/2)x - (1/2)x = xlnx
This confirms that our integration is correct.
Exploring Related Integrals
Understanding the integral of xlnx provides a foundation for solving similar integrals. Let's explore some related examples:
1. Integral of x²lnx
Following a similar approach using integration by parts:
u = lnx=>du = (1/x)dxdv = x²dx=>v = (1/3)x³
Applying the formula:
∫x²lnx dx = (1/3)x³lnx - (1/3)∫x² dx = (1/3)x³lnx - (1/9)x³ + C
2. Integral of xⁿlnx (where n is a constant)
Generalizing the pattern, we can solve the integral of xⁿlnx using integration by parts:
u = lnx=>du = (1/x)dxdv = xⁿdx=>v = xⁿ⁺¹/(n+1)(assuming n ≠ -1)
∫xⁿlnx dx = dx = [xⁿ⁺¹lnx]/(n+1) - [xⁿ⁺¹/(n+1)²] + C
3. Integral of ln x
This seemingly simpler integral can also be solved using integration by parts with a clever trick:
u = lnx=>du = (1/x)dxdv = dx=>v = x
∫lnx dx = xlnx - ∫x(1/x)dx = xlnx - ∫dx = xlnx - x + C
Applications of the Integral of xlnx
The integral of xlnx, and its variations, appears in various applications across different fields:
-
Probability and Statistics: In probability distributions, such integrals often arise when calculating expected values or moments. For example, calculating the expectation of certain continuous random variables.
-
Physics and Engineering: Integrals of this form can appear in solving differential equations that model physical phenomena. For example, in problems involving heat transfer or fluid dynamics.
-
Economics: Similar integrals might be used in economic modeling, particularly concerning growth or decay functions.
-
Computer Science: The integral can appear in the analysis of algorithms' complexity or efficiency.
Advanced Techniques and Considerations
For more complex integrals involving logarithmic and polynomial functions, more advanced techniques such as substitution or partial fraction decomposition may be needed in conjunction with integration by parts. Mastering these techniques is crucial for handling a broader range of integration problems.
Conclusion
The integral of xlnx, while seemingly basic, provides a valuable demonstration of integration by parts and its applications. By understanding the step-by-step process and related techniques, one can effectively tackle a wide range of integration problems involving logarithmic and polynomial functions. The applications of this integral extend far beyond the realm of pure mathematics, proving its significance in various scientific and engineering disciplines. Remember to always verify your solutions by differentiating the result, and don't hesitate to explore and master advanced integration techniques to further enhance your problem-solving abilities.
Latest Posts
Latest Posts
-
How Many Ft Is 59 Inches
Apr 06, 2025
-
40 In Is How Many Feet
Apr 06, 2025
-
What Is 190 Lb In Kilograms
Apr 06, 2025
-
4000 Grams Is How Many Pounds
Apr 06, 2025
-
How Tall Is 1 87 Meters In Feet
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Is The Integral Of Xlnx . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
