What Is 15 Percent Of 120
Greels
Mar 28, 2025 · 5 min read
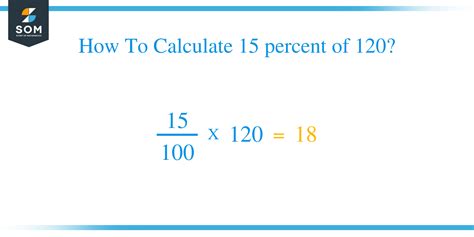
Table of Contents
What is 15 Percent of 120? A Deep Dive into Percentages and Their Applications
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from everyday finances to complex scientific analyses. Understanding how to determine a percentage of a number is crucial for making informed decisions, budgeting effectively, and comprehending data presented in percentage form. This comprehensive guide will explore how to calculate 15 percent of 120, delve into the underlying principles of percentage calculations, and showcase various real-world applications to solidify your understanding.
Understanding Percentages: A Foundational Concept
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "per hundred" or "out of one hundred." For instance, 15% represents 15 out of 100, which can be expressed as the fraction 15/100 or the decimal 0.15.
Percentages are versatile tools used to express proportions, ratios, and rates of change. They are prevalent in numerous contexts, including:
- Finance: Calculating interest rates, discounts, taxes, and profit margins.
- Statistics: Representing data distributions, probabilities, and error margins.
- Science: Expressing concentrations, yields, and uncertainties in measurements.
- Everyday Life: Understanding sales, tips, and changes in prices or quantities.
Calculating 15 Percent of 120: Three Methods
There are several methods to determine 15 percent of 120. Let's explore three common approaches:
Method 1: Using the Decimal Equivalent
This is arguably the most straightforward method. We convert the percentage to its decimal equivalent and then multiply it by the given number.
-
Convert the percentage to a decimal: 15% is equivalent to 0.15 (divide 15 by 100).
-
Multiply the decimal by the number: 0.15 * 120 = 18
Therefore, 15 percent of 120 is 18.
Method 2: Using the Fraction Equivalent
We can also use the fractional equivalent of 15%.
-
Express the percentage as a fraction: 15% is equivalent to 15/100.
-
Simplify the fraction (optional): 15/100 simplifies to 3/20.
-
Multiply the fraction by the number: (3/20) * 120 = 18
Again, we find that 15 percent of 120 is 18.
Method 3: Using Proportions
This method uses the concept of ratios and proportions.
-
Set up a proportion: We can set up a proportion where x represents 15% of 120:
x/120 = 15/100
-
Solve for x: Cross-multiply and solve for x:
100x = 15 * 120 100x = 1800 x = 1800/100 x = 18
Once more, the answer is 18.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in many real-life situations. Let's examine some examples:
Finance and Budgeting
-
Sales Tax: If a product costs $120 and the sales tax is 15%, the tax amount is 15% of $120, which is $18. The total cost would be $120 + $18 = $138.
-
Discounts: A store offers a 15% discount on a $120 item. The discount amount is $18, and the final price is $120 - $18 = $102.
-
Interest Calculations: If you invest $120 and earn 15% annual interest, you would earn $18 in interest after one year.
-
Investment Returns: Understanding percentage returns on investments is crucial for financial planning and decision-making.
-
Loan Payments: Calculating monthly loan payments often involves percentage calculations of the principal amount and interest rates.
Statistics and Data Analysis
-
Data Representation: Percentages are widely used in graphs and charts to visually represent proportions of data sets. For example, a pie chart might show that 15% of survey respondents prefer a particular product.
-
Probability and Odds: In probability, percentages are used to express the likelihood of an event occurring. A 15% chance of rain, for example, means there's a 15/100 probability of rain.
-
Error Margins: In statistical analysis, percentages are used to represent the margin of error in surveys and experiments.
Science and Engineering
-
Concentration Calculations: In chemistry, percentages are frequently used to express the concentration of solutions. For instance, a 15% solution of salt in water means that 15 grams of salt are dissolved in 100 grams of solution.
-
Yield Calculations: In chemical reactions and manufacturing processes, percentage yield indicates the efficiency of a reaction or process.
-
Measurement Errors: Percentages are used to quantify measurement errors or uncertainties in scientific experiments.
Advanced Percentage Calculations: Beyond the Basics
While calculating 15% of 120 is relatively straightforward, more complex percentage problems often arise. Here are some scenarios requiring more advanced techniques:
Finding the Original Amount
If you know the percentage and the resulting amount, you can work backward to find the original amount. For example:
"15% of a number is 18. What is the number?"
To solve this:
- Represent the unknown number as 'x'.
- Set up the equation: 0.15x = 18
- Solve for x: x = 18 / 0.15 = 120
Calculating Percentage Increase or Decrease
Percentage changes are crucial for tracking growth or decline in various quantities. The formula is:
[(New Value - Old Value) / Old Value] * 100%
For instance, if a quantity increases from 120 to 138, the percentage increase is:
[(138 - 120) / 120] * 100% = 15%
Compound Interest
Compound interest involves earning interest on both the principal amount and accumulated interest. The formula for compound interest is:
A = P (1 + r/n)^(nt)
Where:
- A = the future value of the investment/loan, including interest
- P = the principal investment amount (the initial deposit or loan amount)
- r = the annual interest rate (decimal)
- n = the number of times that interest is compounded per year
- t = the number of years the money is invested or borrowed for
Conclusion: Mastering Percentage Calculations
Understanding percentage calculations is a valuable skill applicable to numerous aspects of life. While calculating 15 percent of 120 might seem like a simple task, it forms the basis for more complex percentage problems. By grasping the fundamental methods and exploring the diverse applications showcased in this guide, you'll be well-equipped to confidently tackle percentage calculations in various contexts and make informed decisions based on quantitative data. Remember to practice regularly, and you'll quickly master this essential mathematical skill.
Latest Posts
Latest Posts
-
31 Kg Is How Many Pounds
Mar 31, 2025
-
How Much Is 120 Kg In Lbs
Mar 31, 2025
-
How Far Is 1 8 Miles In Km
Mar 31, 2025
-
How Long Is 75 Inches In Feet
Mar 31, 2025
-
Cuanto Es 167 Libras En Kg
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is 15 Percent Of 120 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
