What Is 10 Percent Of 300
Greels
Mar 27, 2025 · 5 min read
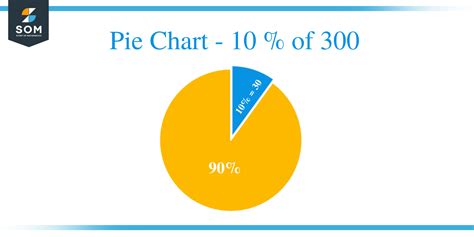
Table of Contents
What is 10 Percent of 300? A Deep Dive into Percentages and Their Applications
Calculating percentages is a fundamental skill applicable across numerous fields, from everyday budgeting to complex financial modeling. Understanding how to determine percentages allows for efficient problem-solving and informed decision-making. This comprehensive guide will not only answer the question, "What is 10 percent of 300?" but will also explore the underlying principles of percentage calculations, provide various methods for solving such problems, and showcase real-world applications to solidify your understanding.
Understanding Percentages
A percentage is simply a fraction expressed as a number out of 100. The term "percent" comes from the Latin "per centum," meaning "out of a hundred." Therefore, 10% means 10 out of 100, which can be written as the fraction 10/100 or the decimal 0.10. This understanding forms the foundation for all percentage calculations.
Calculating 10 Percent of 300: The Direct Method
The most straightforward approach to finding 10 percent of 300 involves converting the percentage to a decimal and multiplying it by the given number. Here's how:
-
Convert the percentage to a decimal: 10% is equal to 0.10 (or simply 0.1).
-
Multiply the decimal by the number: 0.1 * 300 = 30
Therefore, 10 percent of 300 is 30.
Alternative Methods: Exploring Different Approaches
While the direct method is efficient, several other methods can be employed to calculate percentages, providing flexibility depending on the context and individual preferences.
Method 2: Using Fractions
Percentages can be represented as fractions. 10% can be expressed as 10/100, which simplifies to 1/10. To find 10% of 300 using this method:
-
Express the percentage as a fraction: 10% = 1/10
-
Multiply the fraction by the number: (1/10) * 300 = 30
This confirms that 10 percent of 300 is 30. This method is particularly useful when dealing with simpler percentages that can be easily reduced to smaller fractions.
Method 3: Proportion Method
The proportion method leverages the relationship between percentages and ratios. We can set up a proportion to solve for the unknown value:
-
Let 'x' represent 10% of 300.
-
We can set up the proportion: 10/100 = x/300
-
Cross-multiply: 10 * 300 = 100 * x
-
Solve for x: 3000 = 100x => x = 3000/100 = 30
Again, we arrive at the answer: 10 percent of 300 is 30. This method is helpful when visualizing the relationship between the percentage and the whole.
Real-World Applications of Percentage Calculations
The ability to calculate percentages isn't confined to mathematical exercises; it's a crucial skill with widespread real-world applications. Here are just a few examples:
1. Sales and Discounts
Retail stores frequently offer discounts expressed as percentages. Understanding percentages allows consumers to quickly calculate the actual savings on an item. For instance, a 20% discount on a $100 item would result in a savings of $20 ($100 x 0.20 = $20).
2. Taxes and Tips
Calculating sales tax or service tips involves percentage calculations. If the sales tax is 6% on a $50 purchase, the tax amount would be $3 ($50 x 0.06 = $3). Similarly, a 15% tip on a $75 restaurant bill would be $11.25 ($75 x 0.15 = $11.25).
3. Financial Investments and Growth
Percentage calculations are essential in finance. Understanding percentage growth or loss in investments helps investors track performance and make informed decisions. A 5% increase in a $10,000 investment would represent a gain of $500 ($10,000 x 0.05 = $500).
4. Grade Calculations
Many academic systems use percentages to represent grades. If a student scores 85 out of 100 on a test, their grade is 85%. This allows for easy comparison and tracking of academic performance.
5. Data Analysis and Statistics
Percentages are commonly used to represent proportions in data analysis and statistics. For example, if a survey shows that 70% of respondents prefer a certain product, it indicates a significant preference.
Advanced Percentage Calculations and Problem Solving
While calculating 10% of 300 is relatively straightforward, many real-world problems require more complex percentage calculations. Let's explore some common scenarios:
Calculating the Percentage One Number Represents of Another
Suppose you have scored 80 marks out of 100 in a test. To find the percentage, you would divide the marks obtained by the total marks and multiply by 100:
(80/100) * 100% = 80%
Finding the Original Value After a Percentage Increase or Decrease
If a product costs $120 after a 20% increase, we can work backward to find the original price. Let's denote the original price as 'x':
x + 0.20x = $120
1.20x = $120
x = $120 / 1.20 = $100
Therefore, the original price was $100.
Calculating Percentage Change
Percentage change helps measure the difference between two values. The formula is:
[(New Value - Old Value) / Old Value] * 100%
For example, if the price of an item increased from $50 to $60, the percentage change is:
[(60 - 50) / 50] * 100% = 20%
Mastering Percentages: Tips and Tricks
Here are some tips and tricks to improve your percentage calculation skills:
-
Memorize common percentage equivalents: Familiarizing yourself with common fractions and their percentage equivalents (e.g., 1/4 = 25%, 1/2 = 50%, 1/10 = 10%) can significantly speed up calculations.
-
Use mental math strategies: For simpler percentages, you can often calculate them mentally using shortcuts and estimation. For example, 10% of a number is simply the number divided by 10.
-
Practice regularly: Consistent practice is key to mastering percentage calculations. Solve various problems and gradually increase the difficulty level.
-
Utilize online calculators and tools: Numerous online calculators can help with percentage calculations, particularly for more complex problems. However, understanding the underlying principles remains crucial for independent problem-solving.
Conclusion
Calculating percentages is a vital skill with diverse applications in various aspects of life. While finding 10 percent of 300 is a basic calculation, understanding the underlying principles allows you to tackle more complex problems efficiently and confidently. By mastering different calculation methods and practicing regularly, you can develop proficiency in percentages and apply this essential skill to various scenarios, from managing your personal finances to analyzing data and making informed decisions. Remember that consistent practice and a solid grasp of the fundamental concepts are the keys to success.
Latest Posts
Latest Posts
-
70 In Is How Many Feet
Mar 30, 2025
-
How Many Feet Is 1200 Inches
Mar 30, 2025
-
Rewrite Equation In Slope Intercept Form
Mar 30, 2025
-
Cuanto Es 169 Libras En Kilos
Mar 30, 2025
-
How Many Kilometers Are In 26 Miles
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about What Is 10 Percent Of 300 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
