Volumes Of Solids Of Revolution Calculator
Greels
Mar 30, 2025 · 6 min read
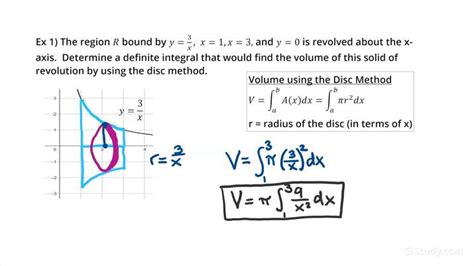
Table of Contents
Volumes of Solids of Revolution Calculator: A Comprehensive Guide
Calculating the volume of a solid of revolution can be a complex mathematical undertaking, often requiring intricate integration techniques. However, with the advent of online calculators and readily available software, this process has become significantly more accessible. This comprehensive guide delves into the intricacies of calculating volumes of solids of revolution, explores the functionalities of online calculators, and provides practical examples to solidify your understanding.
Understanding Solids of Revolution
Before diving into the mechanics of calculation, let's establish a firm understanding of what a solid of revolution is. Imagine taking a two-dimensional curve (a function) and rotating it around an axis (typically the x-axis or y-axis). The three-dimensional shape that results from this rotation is a solid of revolution. Examples include cones, spheres, cylinders, and many more complex shapes.
The volume of these solids can be determined using integral calculus, specifically using the disk method, washer method, or shell method. The choice of method depends on the shape of the curve and the axis of rotation.
The Disk Method
The disk method is applicable when the region is rotated around an axis such that the resulting solid has cross-sections that are disks. This typically happens when the curve is rotated about the x-axis or y-axis, and the region is bounded by the curve and the axis of rotation.
The formula for the disk method is:
V = π ∫<sub>a</sub><sup>b</sup> [f(x)]<sup>2</sup> dx (for rotation about the x-axis)
V = π ∫<sub>c</sub><sup>d</sup> [f(y)]<sup>2</sup> dy (for rotation about the y-axis)
where:
- V represents the volume of the solid.
- a and b (or c and d) are the limits of integration.
- f(x) (or f(y)) is the function defining the curve.
The Washer Method
When the region being rotated has a hole in the middle, the washer method is employed. This occurs when the region is bounded by two curves, and the solid of revolution has cross-sections that resemble washers (disks with holes).
The formula for the washer method is:
V = π ∫<sub>a</sub><sup>b</sup> ([R(x)]<sup>2</sup> - [r(x)]<sup>2</sup>) dx (for rotation about the x-axis)
V = π ∫<sub>c</sub><sup>d</sup> ([R(y)]<sup>2</sup> - [r(y)]<sup>2</sup>) dy (for rotation about the y-axis)
where:
- R(x) (or R(y)) is the outer radius of the washer.
- r(x) (or r(y)) is the inner radius of the washer.
The Shell Method
The shell method offers an alternative approach, particularly useful when the integration becomes significantly simpler using this technique. It considers cylindrical shells as the building blocks of the solid.
The formula for the shell method is:
V = 2π ∫<sub>a</sub><sup>b</sup> x * f(x) dx (for rotation about the y-axis)
V = 2π ∫<sub>c</sub><sup>d</sup> y * f(y) dy (for rotation about the x-axis)
Utilizing Online Volumes of Solids of Revolution Calculators
Online calculators significantly streamline the process of calculating the volume of solids of revolution. These calculators typically require you to input the function, the limits of integration, and the axis of rotation. The calculator then performs the necessary integration and provides the volume.
Key Features to Look for in a Calculator:
- Multiple Methods: A good calculator will support all three methods (disk, washer, and shell) to allow for flexibility based on the problem's complexity.
- Step-by-Step Solutions: Calculators offering step-by-step solutions provide valuable insights into the integration process, aiding in learning and understanding.
- Clear Input Fields: Intuitive input fields for the function, limits of integration, and axis of rotation are essential for user-friendly experience.
- Graphing Capabilities: Some advanced calculators include graphing capabilities, allowing you to visualize the curve and the solid of revolution, further enhancing understanding.
- Handling of Complex Functions: The calculator should be capable of handling a wide variety of functions, including trigonometric, exponential, and logarithmic functions.
Practical Examples
Let's illustrate the process with a couple of examples.
Example 1: Disk Method
Find the volume of the solid generated by rotating the region bounded by y = x² and the x-axis from x = 0 to x = 1 around the x-axis.
Using the disk method:
V = π ∫<sub>0</sub><sup>1</sup> (x²)² dx = π ∫<sub>0</sub><sup>1</sup> x<sup>4</sup> dx = π [x<sup>5</sup>/5]<sub>0</sub><sup>1</sup> = π/5
Example 2: Washer Method
Find the volume of the solid generated by rotating the region bounded by y = x² and y = x from x = 0 to x = 1 around the x-axis.
Using the washer method:
V = π ∫<sub>0</sub><sup>1</sup> [(x)<sup>2</sup> - (x²)<sup>2</sup>] dx = π ∫<sub>0</sub><sup>1</sup> (x² - x<sup>4</sup>) dx = π [x³/3 - x<sup>5</sup>/5]<sub>0</sub><sup>1</sup> = 2π/15
Example 3: Shell Method
Find the volume of the solid generated by rotating the region bounded by y = x and y = x² around the y-axis.
Using the shell method:
V = 2π ∫<sub>0</sub><sup>1</sup> x(x - x²) dx = 2π ∫<sub>0</sub><sup>1</sup> (x² - x³) dx = 2π [x³/3 - x<sup>4</sup>/4]<sub>0</sub><sup>1</sup> = π/6
Beyond Basic Calculations: Advanced Applications
While online calculators handle the core calculations efficiently, understanding the underlying principles and the nuances of different methods is crucial for tackling more complex scenarios. These might include:
- Rotation around lines other than the x or y-axis: This requires adjusting the formulas to account for the distance from the axis of rotation.
- Regions bounded by more than two curves: These scenarios often require careful consideration of the limits of integration and the application of appropriate techniques.
- Solids with non-uniform cross-sections: For solids where the cross-sections are not simple disks or washers, more advanced techniques and potentially numerical integration might be necessary.
Conclusion
Calculating the volume of solids of revolution can be challenging, but online calculators offer a powerful tool to simplify the process. Understanding the different methods – disk, washer, and shell – allows for selecting the most efficient approach for a given problem. While calculators expedite the numerical computation, a firm grasp of the underlying mathematical principles is essential for handling complex problems and applying these concepts to broader areas of mathematics and engineering. Remember to always carefully analyze the problem geometry and select the appropriate method before employing a calculator to ensure accurate results.
Latest Posts
Latest Posts
-
How Many Pounds Is 1500 Kg
Apr 01, 2025
-
How Much Is 20 Ml In Oz
Apr 01, 2025
-
Cuanto Es 220 Libras En Kilos
Apr 01, 2025
-
How Many Inches Is 420 Mm
Apr 01, 2025
-
How Many Miles Is 1300 Km
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Volumes Of Solids Of Revolution Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
