Two Step Equation Solver With Fractions
Greels
Mar 29, 2025 · 5 min read
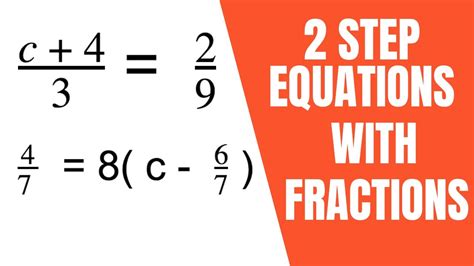
Table of Contents
Two-Step Equations with Fractions: A Comprehensive Guide
Solving equations is a fundamental skill in algebra. While simple equations might seem straightforward, incorporating fractions adds a layer of complexity that can be daunting for many students. This comprehensive guide will walk you through solving two-step equations containing fractions, providing clear explanations, step-by-step examples, and helpful tips to build your confidence and mastery of this important mathematical concept.
Understanding Two-Step Equations
Before diving into fractions, let's review the basic structure of a two-step equation. A two-step equation is an algebraic equation that requires two steps to isolate the variable and solve for its value. The general form looks like this:
ax + b = c
Where:
xis the variable we want to solve for.aandbare constants (numbers).cis a constant on the other side of the equals sign.
To solve this, we perform two operations:
- Undo the addition or subtraction: Add or subtract the constant 'b' from both sides of the equation.
- Undo the multiplication or division: Multiply or divide both sides by the constant 'a'.
Incorporating Fractions: The Challenges
Adding fractions to the mix introduces several potential challenges:
- Finding Common Denominators: Working with unlike fractions necessitates finding a common denominator before adding or subtracting them.
- Reciprocal Multiplication: To eliminate a fractional coefficient (the 'a' in our general form), we utilize the reciprocal. Understanding and applying reciprocals is crucial.
- Simplifying Expressions: The process often involves simplifying fractions, which requires reducing them to their lowest terms.
Step-by-Step Guide to Solving Two-Step Equations with Fractions
Let's tackle some examples to illustrate the process effectively. We'll break down each step meticulously, explaining the rationale behind each action.
Example 1: A Simple Case
Solve for x: (1/2)x + 3 = 7
Step 1: Isolate the term with 'x'
Subtract 3 from both sides of the equation:
(1/2)x + 3 - 3 = 7 - 3
(1/2)x = 4
Step 2: Solve for 'x'
Multiply both sides by the reciprocal of (1/2), which is 2:
2 * (1/2)x = 4 * 2
x = 8
Example 2: Dealing with Unlike Fractions
Solve for y: (2/3)y - (1/6) = (5/2)
Step 1: Find a Common Denominator
The least common denominator for 3, 6, and 2 is 6. Rewrite the equation with a common denominator:
(4/6)y - (1/6) = (15/6)
Step 2: Isolate the term with 'y'
Add (1/6) to both sides:
(4/6)y - (1/6) + (1/6) = (15/6) + (1/6)
(4/6)y = (16/6)
Step 3: Solve for 'y'
Multiply both sides by the reciprocal of (4/6), which is (6/4):
(6/4) * (4/6)y = (16/6) * (6/4)
y = 4
Example 3: A More Complex Scenario
Solve for z: (3/4)z + (2/5) = (7/10)
Step 1: Find a Common Denominator
The least common denominator for 4, 5, and 10 is 20. Rewrite the equation:
(15/20)z + (8/20) = (14/20)
Step 2: Isolate the term with 'z'
Subtract (8/20) from both sides:
(15/20)z + (8/20) - (8/20) = (14/20) - (8/20)
(15/20)z = (6/20)
Step 3: Solve for 'z'
Multiply both sides by the reciprocal of (15/20), which is (20/15):
(20/15) * (15/20)z = (6/20) * (20/15)
z = (6/15)
Step 4: Simplify the Fraction
Reduce the fraction to its lowest terms:
z = (2/5)
Common Mistakes to Avoid
- Incorrectly applying the reciprocal: Remember to multiply by the reciprocal, not just flip the fraction.
- Forgetting to apply the operation to both sides: Always perform the same operation on both sides of the equation to maintain balance.
- Errors in simplifying fractions: Double-check your fraction simplification to ensure accuracy.
- Neglecting to find a common denominator: Adding or subtracting fractions requires a common denominator.
- Arithmetic errors: Carefully check your calculations to minimize errors.
Tips for Success
- Practice regularly: Consistent practice is key to mastering this skill.
- Break down the problem into smaller steps: This makes the process less overwhelming.
- Check your work: Substitute your solution back into the original equation to verify its accuracy.
- Utilize online resources: Many websites and apps offer practice problems and tutorials.
- Seek help when needed: Don't hesitate to ask for assistance from teachers, tutors, or peers.
Advanced Applications and Extensions
The techniques learned here extend to more complex equations. You'll encounter these principles in solving:
- Equations with mixed numbers: Convert mixed numbers to improper fractions before solving.
- Equations with variables on both sides: Use inverse operations to collect variables on one side and constants on the other.
- Inequalities with fractions: Similar steps apply to solving inequalities, but remember to flip the inequality sign if you multiply or divide by a negative number.
- Word problems involving fractions: Translate word problems into algebraic equations before solving.
Conclusion
Solving two-step equations with fractions might seem challenging initially, but with consistent practice and a solid understanding of the underlying principles, you can master this crucial algebraic skill. By breaking down the process into manageable steps, carefully attending to detail, and utilizing the strategies outlined in this guide, you can build confidence and achieve success in tackling even the most complex equations involving fractions. Remember, the key is consistent practice and a willingness to learn from mistakes. Keep practicing, and you'll become proficient in no time!
Latest Posts
Latest Posts
-
How Many Inches Is 165 Cm
Apr 01, 2025
-
What Is The Derivative Of 3x
Apr 01, 2025
-
4 5 Meters Is How Many Feet
Apr 01, 2025
-
What Is 93 Pounds In Kg
Apr 01, 2025
-
How Many Feet Is 195 Cm
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Two Step Equation Solver With Fractions . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
