Solve By Complete The Square Calculator
Greels
Apr 05, 2025 · 6 min read
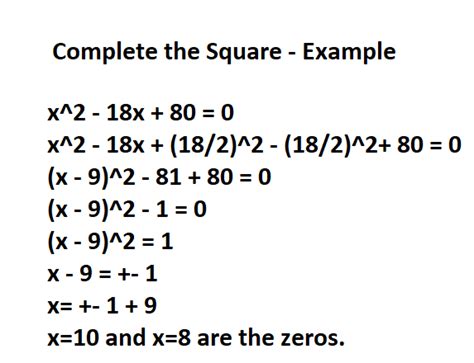
Table of Contents
Solve by Completing the Square Calculator: A Comprehensive Guide
Completing the square is a crucial algebraic technique used to solve quadratic equations, find the vertex of a parabola, and simplify various mathematical expressions. While the process is straightforward, it can be time-consuming and prone to errors, especially with complex equations. This is where a "solve by completing the square calculator" comes in handy. This article will delve deep into the concept of completing the square, explore its applications, and guide you on effectively utilizing a completing the square calculator to solve quadratic equations efficiently and accurately.
Understanding Completing the Square
Before diving into the use of calculators, let's solidify our understanding of the completing the square method. This technique involves manipulating a quadratic equation of the form ax² + bx + c = 0 to transform it into a perfect square trinomial, which can then be easily factored and solved.
The core idea lies in recognizing the structure of a perfect square trinomial: (x + p)² = x² + 2px + p². Notice that the constant term (p²) is the square of half the coefficient of the x term (p). This observation forms the basis of the completing the square method.
Steps involved in completing the square:
-
Ensure the coefficient of x² is 1: If the coefficient of x² (a) is not 1, divide the entire equation by 'a'.
-
Move the constant term to the right side: Isolate the terms containing x on the left side of the equation.
-
Find the value to complete the square: Take half of the coefficient of x (b/2) and square it ((b/2)²). This is the value needed to complete the square.
-
Add the value to both sides: Add this value ((b/2)²) to both sides of the equation to maintain balance.
-
Factor the perfect square trinomial: The left side of the equation will now be a perfect square trinomial, which can be factored as (x + b/2)².
-
Solve for x: Take the square root of both sides, remembering to consider both positive and negative roots. Then solve for x.
Example: Solving a Quadratic Equation by Completing the Square
Let's solve the equation x² + 6x + 5 = 0 using the completing the square method:
-
Coefficient of x² is already 1.
-
Move the constant term: x² + 6x = -5
-
Complete the square: Half of the coefficient of x (6/2 = 3), squared (3² = 9).
-
Add to both sides: x² + 6x + 9 = -5 + 9 => x² + 6x + 9 = 4
-
Factor: (x + 3)² = 4
-
Solve for x: Taking the square root of both sides: x + 3 = ±2. This gives two solutions: x = -1 and x = -5.
The Role of a Solve by Completing the Square Calculator
Manual completion of the square can be tedious, especially when dealing with fractions, decimals, or large numbers. A "solve by completing the square calculator" streamlines this process by automating the steps. These calculators typically require you to input the coefficients a, b, and c from your quadratic equation (ax² + bx + c = 0). The calculator then performs the completing the square process and presents the solution, often showing the intermediate steps for better understanding.
Benefits of Using a Completing the Square Calculator
-
Increased Accuracy: Manual calculations are susceptible to errors, particularly in the middle steps. A calculator eliminates this risk, ensuring accurate results.
-
Time Efficiency: Completing the square manually can be time-consuming, especially with complicated equations. A calculator significantly reduces the time spent on calculations, allowing you to focus on other aspects of problem-solving.
-
Enhanced Learning: While calculators provide solutions, many also show the step-by-step process. This allows students to learn the method and verify their own workings, improving their understanding of the concept.
-
Handling Complex Equations: Calculators can effortlessly handle equations with fractions, decimals, and irrational coefficients, tasks that can be challenging to perform manually without errors.
-
Verification of Solutions: After manually completing the square, you can use the calculator to verify the accuracy of your solution. This is valuable for building confidence and identifying potential mistakes in your calculations.
Choosing and Using a Completing the Square Calculator
When selecting a calculator, prioritize user-friendliness and clarity. Look for calculators with:
-
Clear Input Fields: The calculator should have well-defined input fields for coefficients a, b, and c.
-
Step-by-Step Solutions: The ability to see the detailed steps is crucial for learning and understanding.
-
Multiple Solution Handling: The calculator should correctly handle equations with two distinct real roots, one repeated root, or no real roots (complex roots).
-
Error Handling: A robust calculator will provide informative error messages if you input incorrect data or an unsolvable equation.
Applications of Completing the Square Beyond Solving Quadratic Equations
The completing the square technique isn't limited to solving quadratic equations. It finds applications in various areas of mathematics, including:
-
Finding the Vertex of a Parabola: The completed square form of a quadratic equation (in the form a(x - h)² + k = 0) directly reveals the vertex of the parabola at (h, k). This is incredibly useful in graphing parabolas and understanding their properties.
-
Simplifying Expressions: Completing the square can simplify complex algebraic expressions, making them easier to manipulate and analyze.
-
Integrating Functions: In calculus, completing the square is a valuable tool in solving certain integrals. It helps transform integrands into forms that are easily integrable.
-
Solving other types of equations: While primarily associated with quadratic equations, the core principle of completing the square can be applied to other types of equations, offering an alternative approach to solving.
Advanced Techniques and Considerations
-
Complex Roots: When the discriminant (b² - 4ac) is negative, the quadratic equation has no real roots but two complex conjugate roots. A complete understanding of completing the square allows you to handle these scenarios and understand the nature of the solutions.
-
Degenerate Cases: In certain cases, the quadratic equation might degenerate into a linear equation. A robust completing the square calculator should be able to handle these degenerate cases correctly.
-
Applications in Geometry: The method finds applications in various geometric problems involving circles, parabolas, ellipses, and hyperbolas. Completing the square can be essential in deriving equations and simplifying geometric relationships.
Conclusion: Empowering Mathematical Problem Solving
The "solve by completing the square calculator" is a powerful tool that enhances mathematical problem-solving capabilities. While understanding the underlying concepts of completing the square is essential, utilizing a calculator boosts accuracy, efficiency, and understanding. It allows individuals to focus on the conceptual aspects of the problem rather than being bogged down by tedious calculations. By effectively leveraging the strengths of both manual calculation and computational tools, you can significantly improve your problem-solving skills and deepen your mathematical understanding. Remember to select a calculator that prioritizes clarity, accuracy, and step-by-step guidance for optimal learning and application.
Latest Posts
Latest Posts
-
How Many Feet In 240 Inches
Apr 05, 2025
-
1500 Kg Is How Many Pounds
Apr 05, 2025
-
How Many Grams Is 4 5 Oz
Apr 05, 2025
-
How Tall Is 203 Cm In Feet
Apr 05, 2025
-
3x 8 2x 3 6 2x 5
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Solve By Complete The Square Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
