Multiplication And Division Of Rational Numbers Calculator
Greels
Mar 31, 2025 · 6 min read
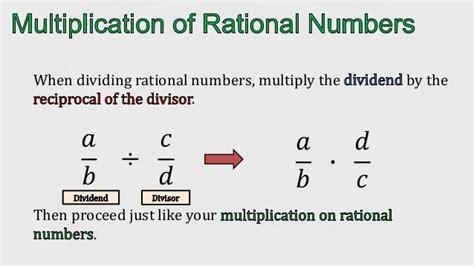
Table of Contents
Multiplication and Division of Rational Numbers Calculator: A Comprehensive Guide
The ability to perform calculations with rational numbers is a fundamental skill in mathematics. Rational numbers, which include integers and fractions, form the backbone of many algebraic and arithmetic operations. While manual calculation is possible, utilizing a multiplication and division of rational numbers calculator can significantly streamline the process, improve accuracy, and save valuable time. This comprehensive guide will delve into the intricacies of rational number calculations, explaining the concepts involved and demonstrating the advantages of using a calculator. We'll also explore different approaches to solving these problems and discuss the practical applications of this essential skill.
Understanding Rational Numbers
Before diving into the mechanics of calculating with rational numbers, let's solidify our understanding of what they are. A rational number is any number that can be expressed as the quotient or fraction p/q of two integers, where p is the numerator and q is the non-zero denominator. This means that any number that can be written as a fraction, where both the numerator and the denominator are whole numbers (and the denominator isn't zero), is a rational number.
Examples of rational numbers include:
- Integers: Numbers like -3, 0, 5, 100 are all rational numbers because they can be expressed as fractions (e.g., -3/1, 0/1, 5/1, 100/1).
- Fractions: Numbers like 1/2, 3/4, -2/5, 7/8 are all rational numbers in their simplest form.
- Terminating Decimals: Decimals that end, such as 0.25 (which is 1/4), 0.75 (which is 3/4), and 0.125 (which is 1/8), are rational numbers.
- Repeating Decimals: Decimals that have a repeating pattern, such as 0.333... (which is 1/3) and 0.142857142857... (which is 1/7), are also rational numbers.
Multiplication of Rational Numbers
Multiplying rational numbers is a straightforward process. To multiply two rational numbers, you simply multiply the numerators together and multiply the denominators together. This can be expressed as:
(a/b) * (c/d) = (a * c) / (b * d)
Example:
Let's multiply 2/3 and 4/5:
(2/3) * (4/5) = (2 * 4) / (3 * 5) = 8/15
Simplifying Fractions:
After multiplying, it's crucial to simplify the resulting fraction to its lowest terms. This involves finding the greatest common divisor (GCD) of the numerator and the denominator and dividing both by it. A multiplication and division of rational numbers calculator will typically handle this simplification automatically.
Example of Simplification:
Let's multiply 6/8 and 2/3:
(6/8) * (2/3) = (6 * 2) / (8 * 3) = 12/24
The GCD of 12 and 24 is 12. Dividing both the numerator and denominator by 12 simplifies the fraction to 1/2.
Division of Rational Numbers
Dividing rational numbers is similar to multiplication but involves an extra step. To divide one rational number by another, you multiply the first fraction by the reciprocal of the second fraction. The reciprocal of a fraction is simply the fraction flipped upside down (numerator and denominator swapped). This can be expressed as:
(a/b) / (c/d) = (a/b) * (d/c) = (a * d) / (b * c)
Example:
Let's divide 2/3 by 4/5:
(2/3) / (4/5) = (2/3) * (5/4) = (2 * 5) / (3 * 4) = 10/12
Simplifying this fraction by dividing both numerator and denominator by their GCD (2) gives 5/6.
Using a Multiplication and Division of Rational Numbers Calculator
A dedicated calculator, either online or a software application, can significantly ease the process of multiplying and dividing rational numbers. These calculators often incorporate the following features:
- Input Flexibility: They accept input in various formats, including fractions (e.g., 1/2, 3/4), mixed numbers (e.g., 1 1/2), and even decimals (though decimals might require conversion to fractions for accurate rational number operations).
- Automatic Simplification: The calculator automatically simplifies the resulting fraction to its lowest terms, eliminating the need for manual simplification.
- Step-by-Step Solutions: Some advanced calculators provide step-by-step solutions, which is immensely helpful for learning and understanding the process. This is particularly useful for educational purposes.
- Error Handling: They usually include error handling for scenarios like division by zero.
- Multiple Operations: Many calculators allow you to perform multiple multiplications and divisions in a single calculation.
Practical Applications of Rational Number Calculations
The ability to perform calculations with rational numbers is crucial in various real-world applications:
- Cooking and Baking: Recipes often involve fractions, and accurately calculating ingredient amounts is vital for successful results. A rational number calculator can be incredibly handy in scaling recipes up or down.
- Construction and Engineering: Precise measurements and calculations are paramount in construction and engineering projects. Rational numbers are frequently used in blueprint interpretations and material calculations.
- Finance and Accounting: Dealing with monetary values, interest rates, and financial ratios often requires manipulating rational numbers.
- Science and Physics: Many scientific formulas and calculations rely heavily on rational numbers, making a calculator a valuable tool in scientific work.
- Everyday Life: From splitting bills fairly among friends to measuring ingredients for a recipe, rational numbers are part of our everyday mathematical experiences.
Beyond Basic Calculations: Advanced Features of Rational Number Calculators
Some advanced calculators offer features beyond basic multiplication and division:
- Support for Mixed Numbers: These calculators handle calculations involving mixed numbers (e.g., 2 1/2) seamlessly.
- Handling of Negative Numbers: They accurately manage calculations involving both positive and negative rational numbers.
- Least Common Multiple (LCM) and Greatest Common Divisor (GCD) Calculations: Many calculators include functionalities to compute the LCM and GCD, useful in simplifying fractions and solving more complex problems.
- Conversion Between Fractions and Decimals: These features allow for easy conversions between fractional and decimal representations of rational numbers.
- Order of Operations: Advanced calculators correctly apply the order of operations (PEMDAS/BODMAS) when presented with multiple operations in a single expression.
Choosing the Right Calculator
The best calculator for you will depend on your specific needs. Consider the following:
- Ease of Use: Opt for a calculator with a user-friendly interface, especially if you're not comfortable with complex mathematical software.
- Functionality: Ensure the calculator offers the features you require, such as support for mixed numbers, simplification, and step-by-step solutions.
- Accessibility: Choose a calculator that's easily accessible on your preferred device (computer, smartphone, or tablet).
Conclusion: Mastering Rational Number Calculations with Technology
The ability to confidently work with rational numbers is a cornerstone of mathematical literacy. While manual calculations are valuable for understanding the underlying concepts, leveraging a multiplication and division of rational numbers calculator can significantly improve efficiency, accuracy, and overall comprehension. By understanding the principles of rational number operations and using the right tools, you'll be well-equipped to tackle a wide range of mathematical problems and apply these skills to various real-world scenarios. The convenience and power offered by these calculators make them invaluable assets for students, professionals, and anyone who regularly works with fractions and rational numbers. Remember to choose a calculator that meets your specific requirements and enhances your mathematical capabilities.
Latest Posts
Latest Posts
-
Cuanto Es 172 Libras En Kilos
Apr 02, 2025
-
How Many Inches Is 0 55 Feet
Apr 02, 2025
-
How Much Is 300 Miles In Kilometers
Apr 02, 2025
-
How Many Feet Is 35 In
Apr 02, 2025
-
How Long Is 100 Cm In Feet
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Multiplication And Division Of Rational Numbers Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
