Integral Of X 2 Ln X
Greels
Apr 02, 2025 · 5 min read
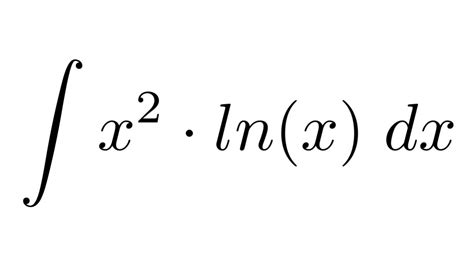
Table of Contents
Definite and Indefinite Integrals of x²lnx: A Comprehensive Guide
The integral of x²lnx is a classic example of an integral requiring integration by parts. This seemingly simple integral holds significant importance in various fields, from calculus exercises to advanced applications in physics and engineering. This comprehensive guide will thoroughly explore both the definite and indefinite integrals of x²lnx, providing detailed explanations and step-by-step solutions. We will delve into the underlying principles, explore alternative approaches, and discuss the significance of this integral in different contexts.
Understanding Integration by Parts
Before tackling the integral of x²lnx, we must understand the fundamental theorem of calculus and, specifically, the technique of integration by parts. Integration by parts is a crucial tool for evaluating integrals of products of functions. It's derived from the product rule for differentiation.
The formula for integration by parts is:
∫u dv = uv - ∫v du
Where:
uandvare functions of x.duanddvare their respective differentials.
The key to successfully applying integration by parts lies in choosing appropriate u and dv. A common mnemonic, "LIATE," can help guide this choice:
- Logarithmic functions
- Inverse trigonometric functions
- Algebraic functions (polynomials)
- Trigonometric functions
- Exponential functions
The order in LIATE suggests prioritizing logarithmic functions as 'u' when present in the integrand, followed by inverse trigonometric, algebraic, and so on. This usually leads to a simpler integral to solve after applying the formula.
Solving the Indefinite Integral of x²lnx
Now, let's apply integration by parts to solve the indefinite integral of x²lnx:
∫x²lnx dx
Following the LIATE rule, we choose:
- u = lnx => du = (1/x) dx
- dv = x² dx => v = (1/3)x³
Substituting these values into the integration by parts formula:
∫x²lnx dx = (1/3)x³lnx - ∫(1/3)x³ * (1/x) dx
Simplifying the integral:
∫x²lnx dx = (1/3)x³lnx - (1/3)∫x² dx
Now, we can easily integrate x²:
∫x² dx = (1/3)x³ + C (where C is the constant of integration)
Therefore, the indefinite integral of x²lnx is:
∫x²lnx dx = (1/3)x³lnx - (1/9)x³ + C
Verifying the Solution Through Differentiation
To verify our solution, we can differentiate the result and see if we get back the original integrand, x²lnx. This is a crucial step in confirming the accuracy of our integration.
Let's differentiate (1/3)x³lnx - (1/9)x³ + C:
d/dx [(1/3)x³lnx - (1/9)x³ + C] = (x²lnx) + (1/3)x³(1/x) - (1/3)x²
Simplifying, we get:
x²lnx + (1/3)x² - (1/3)x² = x²lnx
As we can see, the derivative matches the original integrand, confirming the accuracy of our solution for the indefinite integral.
Solving the Definite Integral of x²lnx
Let's now consider the definite integral of x²lnx within specific limits, say from a to b, where 0 < a < b. The definite integral represents the area under the curve of x²lnx between these limits.
The definite integral is calculated as follows:
∫<sub>a</sub><sup>b</sup> x²lnx dx = [(1/3)x³lnx - (1/9)x³]<sub>a</sub><sup>b</sup>
This involves substituting the upper limit (b) and the lower limit (a) into the indefinite integral and subtracting the results:
∫<sub>a</sub><sup>b</sup> x²lnx dx = [(1/3)b³lnb - (1/9)b³] - [(1/3)a³lna - (1/9)a³]
This expression gives the exact value of the definite integral between the specified limits a and b. Remember that the natural logarithm is only defined for positive values of x, so both a and b must be positive.
Exploring Alternative Approaches
While integration by parts is the most straightforward method, it's beneficial to know that other approaches can sometimes be used, depending on the context and the level of mathematical sophistication. These methods might involve more complex techniques or transformations. For instance, one could potentially explore the use of series expansion, although this often leads to more complicated calculations. However, understanding the limitations of alternative approaches compared to the elegance and efficiency of integration by parts highlights the power of this fundamental technique.
Applications of the Integral of x²lnx
The integral of x²lnx, although seemingly a simple calculus problem, finds applications in various fields. It frequently appears in:
- Probability and Statistics: In probability density functions and calculations involving moments.
- Physics: In solving differential equations that arise in modeling physical phenomena, especially those involving logarithmic relationships.
- Engineering: In areas involving integration and modeling of various systems where logarithmic functions are involved.
- Economics: Certain economic models utilize logarithmic functions to represent growth or utility functions. The integral might appear in calculations related to these models.
- Numerical Analysis: The integral is also useful in numerical integration techniques where approximations of definite integrals are needed. This is especially crucial when analytical solutions are difficult to obtain.
Addressing Potential Challenges and Common Errors
Several common mistakes students often make when evaluating this integral include:
- Incorrect application of integration by parts: Choosing inappropriate
uanddvcan lead to a more complicated integral that is difficult, if not impossible, to solve. Remembering the LIATE rule can help avoid this pitfall. - Errors in differentiation or simplification: Careless mistakes in differentiation or algebraic simplification can lead to incorrect final answers. Checking each step carefully is crucial.
- Forgetting the constant of integration (C) in indefinite integrals: This constant is essential when dealing with indefinite integrals, as it accounts for the infinite family of antiderivatives.
Conclusion: Mastering the Integral of x²lnx
This guide has provided a comprehensive exploration of the integral of x²lnx, covering both definite and indefinite forms. We’ve emphasized the importance of integration by parts, illustrated its application step-by-step, and shown how to verify the solution through differentiation. Understanding this integral is crucial not only for mastering calculus techniques but also for its applications in various scientific and engineering fields. By carefully selecting the u and dv and diligently checking each step, one can confidently tackle this and similar integration problems. Furthermore, appreciating its relevance in various applications reinforces the practical significance of this seemingly basic calculus problem. The ability to solve such integrals accurately and efficiently underscores a strong foundation in calculus and its broader applicability.
Latest Posts
Latest Posts
-
What Is 13 Days From Today
Apr 03, 2025
-
What Day Was 300 Days Ago
Apr 03, 2025
-
How Many Feet Are In 110 Inches
Apr 03, 2025
-
How Many Inches Is 320 Mm
Apr 03, 2025
-
What Day Is It In 9 Days
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Integral Of X 2 Ln X . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
