Integral Of 1 Y 2 1
Greels
Apr 06, 2025 · 4 min read
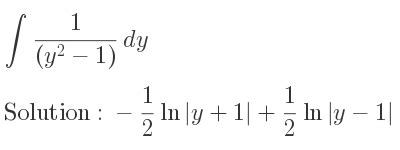
Table of Contents
The Definite Integral: Exploring ∫₁² (1/y²) dy
The seemingly simple definite integral, ∫₁² (1/y²) dy, offers a rich opportunity to explore fundamental concepts in calculus, specifically integration. This article delves deep into the process of solving this integral, explaining the techniques involved, and highlighting its broader applications within mathematics and various scientific fields.
Understanding the Problem: ∫₁² (1/y²) dy
Before diving into the solution, let's break down what the integral represents. The expression ∫₁² (1/y²) dy denotes a definite integral, meaning we are calculating the area under the curve of the function f(y) = 1/y² between the limits y = 1 and y = 2. The function 1/y², or y⁻², is a simple power function, and its integral can be found using the power rule of integration.
The Power Rule of Integration: A Crucial Tool
The power rule of integration is a cornerstone of integral calculus. It states that the integral of xⁿ (where n ≠ -1) is (xⁿ⁺¹)/(n+1) + C, where C is the constant of integration. In our case, n = -2, so we can apply the power rule directly.
Applying the Power Rule to 1/y²
Applying the power rule to our function, 1/y² or y⁻², we get:
∫y⁻² dy = (y⁻²⁺¹)/(-2 + 1) + C = (y⁻¹)/(-1) + C = -1/y + C
This is the indefinite integral, meaning it represents a family of functions whose derivatives are 1/y². The '+ C' signifies the constant of integration, acknowledging that the derivative of a constant is zero.
Evaluating the Definite Integral: Finding the Area
To find the definite integral ∫₁² (1/y²) dy, we need to evaluate the indefinite integral at the upper and lower limits of integration (2 and 1, respectively) and then subtract the results.
-
Evaluate at the upper limit (y = 2):
-1/2 + C
-
Evaluate at the lower limit (y = 1):
-1/1 + C = -1 + C
-
Subtract the lower limit result from the upper limit result:
(-1/2 + C) - (-1 + C) = -1/2 + C + 1 - C = 1/2
Therefore, the definite integral ∫₁² (1/y²) dy equals 1/2. This value represents the area under the curve of f(y) = 1/y² between y = 1 and y = 2.
Visualizing the Integral: A Geometric Interpretation
It's helpful to visualize this result graphically. The function f(y) = 1/y² is a hyperbola that lies entirely above the y-axis for positive values of y. The integral calculates the area enclosed between the curve, the y-axis, and the horizontal lines y = 1 and y = 2. This area is a finite, positive quantity equal to 1/2.
Applications of Definite Integrals: Beyond the Basics
The seemingly simple integral ∫₁² (1/y²) dy has broader significance. Definite integrals are fundamental tools across various scientific and engineering disciplines:
1. Physics: Calculating Work and Displacement
In physics, definite integrals are used to calculate the work done by a force over a distance or the displacement of an object under varying acceleration. The integral represents the accumulation of small contributions over a continuous interval.
2. Engineering: Determining Area and Volume
Engineers use definite integrals to calculate areas of irregular shapes and volumes of complex objects. This is crucial in structural analysis, fluid mechanics, and other engineering fields.
3. Probability and Statistics: Computing Expected Values
In probability and statistics, definite integrals are vital for calculating expected values of continuous random variables. The integral represents the weighted average of all possible outcomes, weighted by their probabilities.
4. Economics: Modeling Consumer Surplus and Producer Surplus
In economics, definite integrals can be used to model consumer surplus (the difference between what consumers are willing to pay and what they actually pay) and producer surplus (the difference between what producers receive and what they are willing to accept).
5. Computer Science: Numerical Integration
In computer science, numerical integration techniques (approximating definite integrals using numerical methods) are essential for solving various problems involving complex functions or data sets where analytical solutions are not readily available.
Further Exploration: Related Integrals and Concepts
This problem serves as a stepping stone to more complex integral calculations. Understanding this basic example provides a strong foundation for tackling more challenging integrals involving:
- More complex functions: Integrals involving trigonometric functions, exponential functions, or logarithmic functions.
- Techniques of integration: Methods like integration by substitution (u-substitution), integration by parts, partial fraction decomposition, and trigonometric substitution.
- Improper integrals: Integrals with infinite limits of integration or integrands with singularities.
- Multiple integrals: Integrals involving multiple variables, used to compute volumes, surface areas, and other multi-dimensional quantities.
Conclusion: Mastery Through Practice
The solution of ∫₁² (1/y²) dy, while seemingly straightforward, provides a valuable entry point into the vast world of integral calculus. The power rule, the concept of definite integrals, and their widespread applications across various fields are all highlighted by this seemingly simple problem. Consistent practice and exploration of more complex integrals will solidify your understanding and build your proficiency in this essential area of mathematics. Remember, the key to mastering calculus lies in understanding the underlying concepts and applying them through consistent practice and problem-solving.
Latest Posts
Latest Posts
-
Cuanto Es 62 Kilos En Libras
Apr 07, 2025
-
How Many Hours In 1000 Minutes
Apr 07, 2025
-
How Many Kg Is 168 Pounds
Apr 07, 2025
-
How Many Kg Is 90 Lbs
Apr 07, 2025
-
How Many Grams Are In 7 Kilograms
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about Integral Of 1 Y 2 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
