Integral Of 1 3 X 2
Greels
Apr 01, 2025 · 5 min read
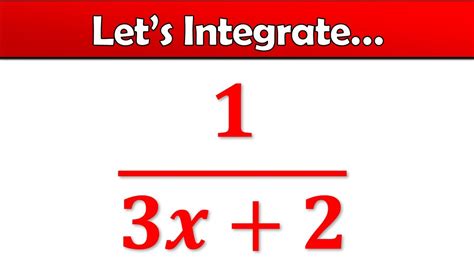
Table of Contents
Decoding the Integral of 1/(3x²): A Comprehensive Guide
The integral of 1/(3x²) might seem deceptively simple at first glance, but understanding its nuances reveals crucial concepts in calculus. This comprehensive guide delves into the intricacies of solving this integral, exploring various methods and highlighting key considerations for accuracy and efficiency. We'll move beyond the basic solution to examine the broader implications and applications of this seemingly straightforward problem.
Understanding the Problem:
The core of the problem lies in evaluating the definite or indefinite integral of the function f(x) = 1/(3x²). In simpler terms, we are seeking the antiderivative of this function. This involves finding a function F(x) such that its derivative, F'(x), equals 1/(3x²).
Method 1: Direct Integration using Power Rule
The most straightforward approach involves manipulating the function to apply the power rule of integration. Remember, the power rule states that the integral of x<sup>n</sup> dx = (x<sup>n+1</sup>)/(n+1) + C, where 'n' is any real number except -1, and 'C' is the constant of integration.
-
Rewrite the function: We can rewrite 1/(3x²) as (1/3)x<sup>-2</sup>. This form is crucial for applying the power rule.
-
Apply the power rule: Now, we integrate:
∫(1/3)x<sup>-2</sup> dx = (1/3) ∫x<sup>-2</sup> dx
Using the power rule: (1/3) * (x<sup>-2+1</sup>)/(-2+1) + C = (1/3) * (x<sup>-1</sup>)/(-1) + C
-
Simplify: This simplifies to -1/(3x) + C.
Therefore, the indefinite integral of 1/(3x²) is -1/(3x) + C. The constant of integration, 'C', is essential because the derivative of any constant is zero. Thus, infinitely many functions can have the same derivative.
Method 2: U-Substitution (for advanced understanding)
While the power rule is the most efficient method for this specific integral, understanding u-substitution provides a valuable tool for tackling more complex problems. Though unnecessary here, let's illustrate the process:
-
Choose a u-substitution: Let's let u = x. Then du = dx. This substitution doesn't significantly simplify the problem in this case, but it demonstrates the method.
-
Rewrite the integral: The integral becomes: ∫(1/3)u<sup>-2</sup> du
-
Apply the power rule: This is identical to step 2 in Method 1, leading to the same result: -1/(3u) + C
-
Substitute back: Finally, substitute x back for u: -1/(3x) + C.
Understanding the Constant of Integration (C)
The constant of integration, 'C', is a crucial element of indefinite integrals. It represents the family of functions that share the same derivative. For example:
- -1/(3x) + 5
- -1/(3x) - 2
- -1/(3x) + π
All of these functions have the same derivative: 1/(3x²). The value of 'C' is determined if we are dealing with a definite integral (explained below).
Definite Integrals: Applying Limits of Integration
A definite integral involves calculating the area under a curve between two specified limits of integration. Let's say we want to find the definite integral of 1/(3x²) from x = 1 to x = 2:
-
Evaluate the indefinite integral: We already know this is -1/(3x) + C.
-
Apply the limits of integration: We evaluate the indefinite integral at the upper and lower limits and subtract:
[-1/(3(2)) + C] - [-1/(3(1)) + C]
-
Simplify: Notice that the constant 'C' cancels out. This is always the case with definite integrals. The result simplifies to:
-1/6 + 1/3 = 1/6
Therefore, the definite integral of 1/(3x²) from x = 1 to x = 2 is 1/6.
Important Considerations and Potential Pitfalls
-
Domain Restrictions: The function 1/(3x²) is undefined at x = 0. This means we cannot integrate across this point. Any definite integral must have limits that exclude x = 0.
-
Improper Integrals: If a limit of integration is infinite or approaches a point where the function is undefined, the integral becomes an improper integral. These require special techniques to evaluate.
-
Numerical Methods: For very complex functions, numerical integration methods (like the Trapezoidal Rule or Simpson's Rule) might be necessary to approximate the definite integral.
Applications of this Integral
While this seemingly simple integral might not immediately leap to mind as having profound applications, its underlying principles are fundamental to many areas of physics, engineering, and other scientific fields. The integral of functions similar to 1/(3x²) appears in:
-
Newton's Law of Universal Gravitation: Calculations involving gravitational forces often involve integrals similar in form. These integrals describe the potential energy associated with gravitational fields.
-
Coulomb's Law: Similar to gravity, electrical forces also involve integrals of inverse-square functions, reflecting the principles of potential energy in electric fields.
-
Probability and Statistics: Probability density functions frequently require integration, often involving functions with forms similar to our example.
Expanding Your Understanding:
This integral is a stepping stone to understanding more complex integrations. Mastering the basics, including the power rule, u-substitution, and the concept of the constant of integration, provides a solid foundation for tackling more challenging problems. Further exploration could involve:
-
Integrals involving trigonometric functions: These introduce new techniques and identities.
-
Integration by parts: This powerful method handles integrals of products of functions.
-
Partial fraction decomposition: This technique helps simplify complex rational functions before integration.
Conclusion:
The integral of 1/(3x²) provides a concise yet valuable introduction to integral calculus. Beyond the simple solution, understanding the nuances of the constant of integration, the application of limits in definite integrals, and potential domain restrictions reveals the richness and depth of this mathematical concept. This knowledge serves as a cornerstone for tackling more advanced integration techniques and understanding its significant role in various scientific disciplines. By grasping these fundamentals, you’ll build a strong foundation for future exploration in calculus and its diverse applications.
Latest Posts
Latest Posts
-
97 Inches Is How Many Feet
Apr 02, 2025
-
How Many Inches Is 68 Mm
Apr 02, 2025
-
How Many Cm In 14 Inches
Apr 02, 2025
-
How Many Miles Is 34 Km
Apr 02, 2025
-
How Many Kg Is 133 Pounds
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Integral Of 1 3 X 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
