Integral 1 X 2 3 2
Greels
Apr 02, 2025 · 5 min read
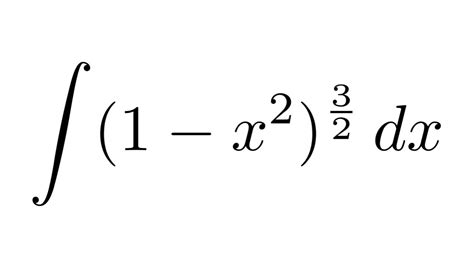
Table of Contents
Decoding the Mystery: A Deep Dive into the Integral of x^(2/3)
The seemingly simple expression, ∫x^(2/3) dx, hides a surprising depth of mathematical concepts. This integral, representing the area under the curve of the function f(x) = x^(2/3), introduces us to fractional exponents, the power rule of integration, and the crucial concept of the constant of integration. This article will dissect this integral step-by-step, exploring its nuances, applications, and broader implications within calculus.
Understanding the Function: f(x) = x^(2/3)
Before embarking on the integration process, let's understand the function itself. f(x) = x^(2/3) is a power function with a fractional exponent. This means the graph is not a simple parabola. The exponent 2/3 indicates a cube root raised to the power of 2, or equivalently, the square of a cube root.
- Domain and Range: The function is defined for all real numbers, meaning its domain is (-∞, ∞). However, the graph's behavior near the origin is noteworthy. Because of the cube root, it is defined for negative x values as well. The range of the function is [0, ∞).
- Graphing the Function: Plotting the function reveals a curve that is steeper near the origin and gradually flattens as x increases. It has a cusp at the origin, meaning it has a sharp point at x=0. This cusp reflects the behavior of the fractional exponent.
- Practical Applications: Functions with fractional exponents appear frequently in various fields. They model phenomena exhibiting non-linear growth or decay, such as:
- Fracture mechanics: Describing the propagation of cracks in materials.
- Fluid dynamics: Modeling certain aspects of fluid flow.
- Economics: Representing certain types of utility functions.
Applying the Power Rule of Integration
The cornerstone of solving this integral is the power rule of integration, a fundamental theorem in calculus. The power rule states:
∫xⁿ dx = (xⁿ⁺¹)/(n+1) + C
Where 'n' is any real number except -1, and 'C' is the constant of integration.
Let's apply this rule to our function, f(x) = x^(2/3):
-
Identify 'n': In our case, n = 2/3.
-
Apply the Power Rule:
∫x^(2/3) dx = (x^(2/3 + 1))/(2/3 + 1) + C
- Simplify the Exponent:
2/3 + 1 = 5/3
Therefore, the integral becomes:
∫x^(2/3) dx = (x^(5/3))/(5/3) + C
- Simplify the Fraction:
Dividing by 5/3 is the same as multiplying by 3/5:
∫x^(2/3) dx = (3/5)x^(5/3) + C
Understanding the Constant of Integration (C)
The constant of integration, 'C', is a crucial aspect of indefinite integrals. It represents an entire family of functions, each differing by a constant value. The derivative of any constant is zero, so when differentiating (3/5)x^(5/3) + C to check our integration, the 'C' disappears.
To find the value of 'C' in a definite integral, you need the limits of integration (the boundaries of the area you're calculating). For example:
∫(from a to b) x^(2/3) dx = = (3/5)b^(5/3) - (3/5)a^(5/3)
Exploring Definite Integrals with Specific Limits
Let's consider a definite integral to solidify our understanding. Let's calculate the area under the curve from x = 1 to x = 8:
∫(from 1 to 8) x^(2/3) dx =
- Substitute Upper Limit:
(3/5)(8)^(5/3) = (3/5)(32) = 19.2
- Substitute Lower Limit:
(3/5)(1)^(5/3) = (3/5)(1) = 0.6
- Subtract:
19.2 - 0.6 = 18.6
Therefore, the area under the curve of f(x) = x^(2/3) from x = 1 to x = 8 is 18.6 square units.
Applications and Extensions
The integral of x^(2/3) has applications beyond simple area calculations. It's a building block for more complex problems. Here are a few examples:
- Volume calculations: Finding the volume of a solid of revolution using the disk or shell method.
- Probability and statistics: Integrating probability density functions to determine probabilities.
- Physics: Calculating work done by a variable force.
- Engineering: Solving differential equations that model various physical systems.
Numerical Methods for Integration
For cases where the antiderivative is not easily obtainable (which is more frequent than you might think!), numerical methods like the Trapezoidal Rule, Simpson's Rule, or more sophisticated techniques are employed to approximate the definite integral. These methods break down the area under the curve into smaller segments, approximating the area of each segment and summing them to achieve an overall approximation. The accuracy of these methods improves as the number of segments increases.
Advanced Considerations
- Complex Numbers: The power rule extends to complex numbers, opening up further mathematical possibilities.
- Generalized Functions: The concept of integration can be extended to include generalized functions (like the Dirac delta function), broadening the applicability of integral calculus.
- Multivariable Calculus: The principles extend to multivariable integration, where we integrate over multiple dimensions.
Conclusion
The seemingly simple integral of x^(2/3) serves as a gateway to a vast landscape of mathematical concepts. It introduces us to the power rule, the significance of the constant of integration, and the practical application of integral calculus across various scientific and engineering disciplines. Mastering this integral strengthens the foundation for tackling more advanced problems in calculus and its numerous applications. Understanding the nuances, such as the behavior of the function and the significance of the constant of integration, empowers a deeper understanding of this fundamental concept and its broader implications within mathematics. The exploration of definite integrals and numerical methods further enhances the practical application and comprehension of this integral, emphasizing its relevance in solving real-world problems.
Latest Posts
Latest Posts
-
Cuanto Es 84 Kilos En Libras
Apr 03, 2025
-
2 5 Pounds Is How Many Grams
Apr 03, 2025
-
What Is 105 Minutes In Hours
Apr 03, 2025
-
164 Cm Is How Many Inches
Apr 03, 2025
-
Cuanto Es 44 Kilos En Libras
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Integral 1 X 2 3 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
