How To Solve A Radical Inequality
Greels
Apr 05, 2025 · 6 min read
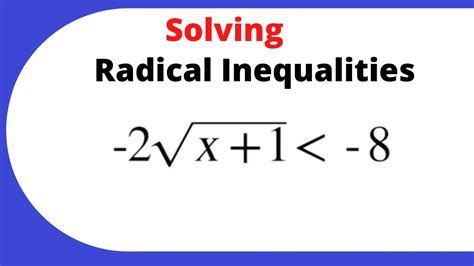
Table of Contents
How to Solve a Radical Inequality: A Comprehensive Guide
Radical inequalities, those involving radical expressions (like square roots, cube roots, etc.), might seem daunting at first, but with a systematic approach, they become manageable. This comprehensive guide will walk you through the process, covering various scenarios and providing ample examples to solidify your understanding. We'll delve into the intricacies of solving radical inequalities, emphasizing the importance of checking solutions and understanding the underlying principles.
Understanding the Fundamentals
Before tackling the complexities of solving radical inequalities, let's review some fundamental concepts:
1. Radical Expressions
A radical expression contains a radical symbol (√), indicating a root. The number inside the radical is called the radicand. The small number (index) outside the radical indicates the type of root (e.g., 2 for square root, 3 for cube root, etc.). If no index is present, it's implicitly a square root.
Examples: √x, ∛(x+2), ⁴√(x²-1)
2. Inequalities
Inequalities represent relationships between quantities where one is greater than, less than, greater than or equal to, or less than or equal to another. We use symbols like < (less than), > (greater than), ≤ (less than or equal to), and ≥ (greater than or equal to) to represent these relationships.
3. Domains of Radical Expressions
A critical consideration when working with radical expressions, especially those with even indices (like square roots), is the domain. The domain is the set of all permissible values for the variable(s) that make the expression defined within the real number system. For even-indexed radicals, the radicand must be non-negative to avoid imaginary numbers. For odd-indexed radicals, there's no such restriction.
Examples:
- √x: The domain is x ≥ 0 (x is greater than or equal to 0)
- ∛x: The domain is all real numbers (-∞, ∞)
- √(x - 2): The domain is x ≥ 2
Solving Radical Inequalities: A Step-by-Step Approach
Solving radical inequalities involves a multi-step process. The exact steps may vary depending on the complexity of the inequality, but the general approach remains consistent:
Step 1: Isolate the Radical Term
The first step is to isolate the radical term on one side of the inequality. This involves performing algebraic operations (addition, subtraction, multiplication, division) to move all other terms to the opposite side. Remember that when multiplying or dividing by a negative number, you must reverse the direction of the inequality sign.
Example: √(x+3) - 2 < 5
Add 2 to both sides: √(x+3) < 7
Step 2: Raise Both Sides to the Power of the Index
Next, raise both sides of the inequality to the power that matches the index of the radical. This eliminates the radical. However, it's crucial to consider the following:
-
Even Indices: When dealing with even indices (square roots, fourth roots, etc.), you must ensure that the expression you're raising to a power is non-negative. If you raise a negative number to an even power, the result is positive. This can lead to extraneous solutions – solutions that satisfy the simplified inequality but not the original one.
-
Odd Indices: Raising both sides to an odd power does not introduce the possibility of extraneous solutions.
Example (Even Index):
√(x+3) < 7
Square both sides: x + 3 < 49
Example (Odd Index):
∛(x-1) > 2
Cube both sides: x - 1 > 8
Step 3: Solve the Resulting Inequality
After eliminating the radical, you'll have a simpler inequality to solve. Use standard algebraic techniques to isolate the variable.
Example:
x + 3 < 49
Subtract 3 from both sides: x < 46
Step 4: Determine the Solution Set Considering the Domain
Remember the domain from Step 1. The solution to the inequality must satisfy both the simplified inequality and the original radical expression's domain.
Example:
We found x < 46. The original inequality was √(x+3) < 7. The domain of √(x+3) is x ≥ -3. To find the intersection of these two conditions (x < 46 and x ≥ -3), we get -3 ≤ x < 46. This is the final solution.
Step 5: Check for Extraneous Solutions (Especially with Even Indices)
This crucial step is often overlooked. Substitute values from your solution set (especially boundary values) back into the original inequality to verify that they satisfy the inequality. If any solution doesn't work, it's an extraneous solution, and you need to remove it from the solution set.
Example: Let's check the boundaries of our solution (-3 ≤ x < 46):
- x = -3: √(-3 + 3) < 7 => 0 < 7 (True)
- x = 45: √(45 + 3) = √48 ≈ 6.93 < 7 (True)
- x = 46: √(46+3) = √49 = 7 which is not less than 7. (False)
Thus, 46 is an extraneous solution.
Advanced Scenarios and Techniques
The process described above provides a solid foundation. However, more complex inequalities may require additional strategies:
1. Inequalities with Multiple Radical Terms
When dealing with inequalities involving multiple radicals, isolate one radical at a time, repeating steps 1-4 for each radical. Pay close attention to the domain of each radical expression.
2. Inequalities Involving Other Operations
Radical inequalities might combine radicals with other operations (e.g., absolute values, polynomials). Treat these problems systematically: tackle the innermost operations first, then proceed to the radicals.
3. Quadratic Inequalities After Removing Radicals
Sometimes, removing the radical leads to a quadratic inequality. Solve these by factoring the quadratic expression and determining the intervals where the inequality holds true. Remember to account for the domain restrictions introduced by the original radical expression.
4. Graphical Solutions
For visualizing the solution set and checking your algebraic work, you can graph both sides of the inequality. The solution set corresponds to the x-values where the graph of one side is above (or below) the graph of the other, depending on the inequality sign. This method is particularly helpful for complex inequalities.
Illustrative Examples
Let's work through a few more examples to reinforce our understanding:
Example 1: Solve √(2x - 1) > 3
-
Isolate the radical: The radical is already isolated.
-
Raise to the power of the index: Square both sides: 2x - 1 > 9
-
Solve the resulting inequality: 2x > 10 => x > 5
-
Consider the domain: The domain of √(2x - 1) is 2x - 1 ≥ 0 => x ≥ 1/2
-
Find the intersection: The solution is x > 5 (since x > 5 already satisfies x ≥ 1/2).
-
Check for extraneous solutions: Checking values greater than 5 confirms the solution.
Example 2: Solve √(x+4) ≤ x
-
Isolate the radical: (This example is a bit trickier as we have 'x' on both sides; it might be tempting to square immediately, but isolation is preferable)
-
Square both sides: x+4 ≤ x²
-
Rearrange into a quadratic: x² - x - 4 ≥ 0
-
Solve the quadratic: Using the quadratic formula, we find the roots to be approximately x ≈ 2.56 and x ≈ -1.56.
-
Determine the intervals: The parabola opens upwards, so the inequality holds for x ≤ -1.56 and x ≥ 2.56.
-
Consider the domain: The domain of √(x+4) is x ≥ -4.
-
Find the intersection: The solution set is x ≥ 2.56 (since x ≥ 2.56 satisfies both conditions, x ≤ -1.56 does not meet the domain restriction, hence it is disregarded).
-
Check for extraneous solutions: Checking values confirms the solution.
Conclusion
Solving radical inequalities requires a structured approach combining algebraic manipulation and careful consideration of the domain. Remember the importance of checking for extraneous solutions, particularly when dealing with even-indexed radicals. By mastering these techniques and practicing regularly, you'll develop the confidence and skills to tackle even the most challenging radical inequalities. Consistent practice and understanding the underlying principles are key to success in this area of algebra. Remember to always check your solutions! A thorough understanding of these concepts will significantly enhance your ability to solve a wide array of mathematical problems.
Latest Posts
Latest Posts
-
How Much Is 200 Grams In Lbs
Apr 06, 2025
-
What Is 15 Percent Of 1200
Apr 06, 2025
-
How Many Pounds Is 15 Grams
Apr 06, 2025
-
70 Millas Por Hora A Km H
Apr 06, 2025
-
18 Centimeters Equals How Many Inches
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about How To Solve A Radical Inequality . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
