Features Of A Rational Graph Calculator
Greels
Apr 02, 2025 · 6 min read
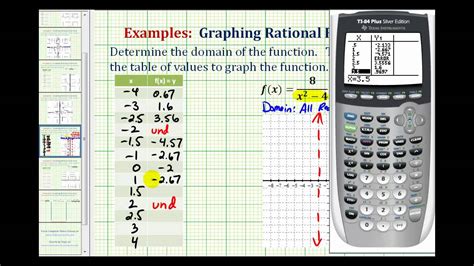
Table of Contents
Unveiling the Power: A Deep Dive into the Features of a Rational Graph Calculator
Rational functions, with their intriguing asymptotes and diverse behaviors, often pose challenges in mathematical analysis. However, the advent of powerful rational graph calculators has revolutionized how we approach these functions, simplifying complex calculations and visualization. This comprehensive guide explores the essential features of a modern rational graph calculator, highlighting their capabilities and demonstrating how they can enhance your mathematical understanding and problem-solving skills.
Beyond Basic Graphing: Unveiling the Advanced Capabilities
While basic graphing calculators can plot functions, a dedicated rational graph calculator offers a wealth of features specifically tailored to handle the nuances of rational expressions. This goes beyond simply displaying the graph; it's about gaining a deep understanding of the function's behavior. Let's delve into the key features:
1. Precise Rational Function Input: Handling Complexity with Ease
The cornerstone of any effective rational graph calculator is its ability to accurately interpret and process rational functions. This involves seamless handling of:
-
Fractions and Polynomials: The calculator should effortlessly accept input in the standard form of a rational function,
P(x)/Q(x), where P(x) and Q(x) are polynomials. It should also correctly handle complex polynomials, including those with high degrees and multiple terms. -
Nested Functions: The ability to input nested rational functions, where rational expressions are embedded within other rational expressions, is crucial for more advanced calculations. The calculator must accurately parse and interpret these nested structures.
-
Symbolic Manipulation: Ideally, the calculator should perform symbolic manipulations on rational functions, simplifying expressions before graphing to ensure accuracy and efficiency. This includes expanding, factoring, and canceling common terms.
Example: A good calculator will readily accept and accurately graph a function like: (3x² + 2x - 1) / (x³ - 4x + 2) without errors or simplifications that alter the function's inherent characteristics.
2. Visualizing Asymptotes: Understanding Function Behavior at Extremes
Asymptotes, the lines that a function approaches but never touches, are fundamental characteristics of rational functions. A high-quality rational graph calculator should provide:
-
Vertical Asymptote Identification: The calculator should automatically detect and display vertical asymptotes, highlighting where the denominator of the rational function equals zero. It should clearly mark these asymptotes on the graph.
-
Horizontal Asymptote Identification: Similarly, the calculator should identify and visually represent horizontal asymptotes, indicating the function's behavior as x approaches positive and negative infinity. The calculator should clearly differentiate between horizontal asymptotes and oblique asymptotes.
-
Oblique Asymptote Identification: For rational functions where the degree of the numerator exceeds the degree of the denominator by one, oblique (slant) asymptotes exist. A sophisticated calculator should detect and display these as well.
-
Interactive Asymptote Exploration: Advanced calculators offer interactive features, allowing users to explore the function's behavior near the asymptotes by zooming in or using cursors to examine values. This interactive exploration fosters a deeper understanding of asymptotic behavior.
Example: The calculator should not only display the vertical asymptote at x = 2 for the function (x+1)/(x-2) but also allow you to investigate the function's behavior as x approaches 2 from both the left and right.
3. Root Finding and Intersection Points: Precise Solutions for Critical Values
Finding roots (x-intercepts) and intersection points between rational functions is often a key step in problem-solving. The features to look for are:
-
Accurate Root Finding: The calculator should accurately locate the x-intercepts of the rational function (where the numerator equals zero). It should handle both real and complex roots, reporting them with sufficient precision.
-
Intersection Point Calculation: The calculator should efficiently compute the intersection points between two or more rational functions. This involves solving a system of equations, which can be complex for rational functions.
-
Iterative Methods Visualization (Optional): Some advanced calculators provide visualizations of iterative methods (like Newton-Raphson) used to find roots, offering a deeper understanding of numerical techniques.
Example: When presented with two rational functions, the calculator should precisely determine the coordinates of their intersection points, clearly marking them on the graph.
4. Advanced Graphing Options: Customization for Clarity and Understanding
A good rational graph calculator offers a range of customization options to enhance the visualization and interpretation of the graph:
-
Zoom and Pan: The ability to zoom in and pan across the graph is crucial for detailed analysis of specific regions of interest, particularly around asymptotes or intersection points.
-
Grid Lines and Axes: Customizable grid lines and axes labels enable users to accurately interpret coordinates and scales.
-
Multiple Function Plotting: Simultaneously plotting multiple rational functions allows for direct comparison and analysis of their behaviors.
-
Trace Functionality: The trace function allows you to move a cursor along the graph and display the coordinates of any point, enabling precise examination of function values.
-
Table Generation: Generating a table of x and y values corresponding to the function's graph allows for numerical analysis alongside the visual representation.
Example: The ability to overlay the graphs of (x+1)/(x-2) and x-1 to clearly visualize their intersection is a valuable feature.
5. Derivatives and Integrals: Exploring Calculus Concepts
Extending beyond basic graphing, a truly advanced rational graph calculator can handle calculus operations:
-
Derivative Calculation: The calculator should be able to compute the derivative of the rational function, either symbolically or numerically. This allows for finding critical points, slopes, and examining the function's rate of change.
-
Integral Calculation (Optional): More sophisticated calculators can calculate definite or indefinite integrals of rational functions, which is essential for area calculations and other calculus applications.
-
Graphical Representation of Derivatives and Integrals: The ability to visually represent the derivative or integral alongside the original function allows for a richer understanding of their relationship.
Example: Being able to display both the graph of a rational function and its derivative allows for a visual understanding of where the function is increasing or decreasing.
6. Error Handling and Robustness: Ensuring Reliable Results
A critical aspect of any mathematical tool is its reliability. A rational graph calculator should:
-
Handle Singularities Gracefully: The calculator should manage cases where the function is undefined (e.g., division by zero) without crashing or producing nonsensical outputs. It should clearly indicate areas where the function is undefined.
-
Provide Clear Error Messages: In the event of an error (e.g., invalid input), the calculator should provide informative and helpful error messages to guide the user towards correcting the input.
-
Numerical Stability: The calculator's algorithms should be numerically stable, ensuring accurate results even with complex or ill-conditioned functions.
Example: When attempting to graph a function with a denominator that is always zero, the calculator should clearly indicate that no graph can be produced rather than generating an error.
The Value Proposition: Why a Dedicated Rational Graph Calculator is Essential
A dedicated rational graph calculator, with its specialized features, offers significant advantages over using general-purpose graphing tools or manual calculations:
-
Enhanced Understanding: The visual representation and interactive features foster a deeper understanding of rational function behavior, asymptotes, and other key properties.
-
Improved Problem Solving: The tools for finding roots, intersection points, and calculating derivatives streamline problem-solving processes, saving time and effort.
-
Accuracy and Efficiency: Automated calculations minimize the risk of human error and drastically increase the speed of analysis.
-
Exploration and Discovery: The interactive nature of these calculators encourages experimentation and exploration, leading to a more intuitive grasp of mathematical concepts.
In conclusion, a sophisticated rational graph calculator is an invaluable tool for students, educators, and anyone working with rational functions. By harnessing its capabilities, you can overcome the challenges associated with these functions and unlock a deeper understanding of their complex and fascinating properties. Choosing a calculator with the features outlined above will significantly enhance your mathematical proficiency and problem-solving abilities.
Latest Posts
Latest Posts
-
Cuanto Es 84 Kilos En Libras
Apr 03, 2025
-
2 5 Pounds Is How Many Grams
Apr 03, 2025
-
What Is 105 Minutes In Hours
Apr 03, 2025
-
164 Cm Is How Many Inches
Apr 03, 2025
-
Cuanto Es 44 Kilos En Libras
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Features Of A Rational Graph Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
