Factoring A Perfect Square Trinomial Calculator
Greels
Apr 06, 2025 · 5 min read
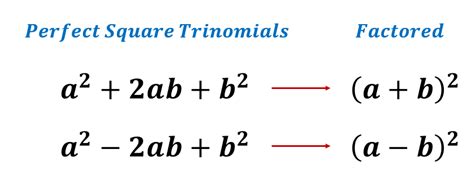
Table of Contents
Factoring a Perfect Square Trinomial Calculator: A Comprehensive Guide
Factoring perfect square trinomials is a fundamental concept in algebra, crucial for solving quadratic equations and simplifying algebraic expressions. While the process itself is relatively straightforward, it can be time-consuming, especially when dealing with complex expressions. This is where a perfect square trinomial calculator comes in handy. This guide will delve deep into the intricacies of perfect square trinomials, explain the process of factoring them manually, and explore the benefits of using a calculator, all while optimizing for SEO and search engine visibility.
Understanding Perfect Square Trinomials
A perfect square trinomial is a polynomial that results from squaring a binomial. It follows a specific pattern that makes it easily identifiable and factorable. The general form is:
a² + 2ab + b² = (a + b)²
or
a² - 2ab + b² = (a - b)²
Let's break down this formula:
- a² and b² represent perfect squares (a number multiplied by itself).
- 2ab represents twice the product of 'a' and 'b'.
Recognizing this pattern is key to factoring these trinomials. For example:
-
x² + 6x + 9 is a perfect square trinomial because it fits the pattern: a = x, b = 3, and 2ab = 2(x)(3) = 6x. Therefore, it factors to (x + 3)².
-
4y² - 12y + 9 is also a perfect square trinomial. Here, a = 2y, b = 3, and 2ab = 2(2y)(3) = 12y. This factors to (2y - 3)².
Factoring Perfect Square Trinomials Manually: A Step-by-Step Approach
While a calculator can expedite the process, understanding the manual method is crucial for a deeper grasp of the underlying mathematical principles. Here's a step-by-step approach:
-
Identify Perfect Squares: Examine the first and last terms of the trinomial. Are they perfect squares? If not, it's not a perfect square trinomial.
-
Check the Middle Term: Take the square root of the first and last terms (these are your 'a' and 'b' values). Multiply 'a' and 'b' by 2. Does this result equal the middle term of the trinomial? If so, you have a perfect square trinomial.
-
Determine the Sign: If the middle term is positive, the factored form will be (a + b)². If the middle term is negative, the factored form will be (a - b)².
-
Write the Factored Form: Substitute your 'a' and 'b' values into the appropriate factored form (a + b)² or (a - b)².
Example: Let's factor 9x² + 24x + 16 manually.
-
Perfect Squares: √9x² = 3x and √16 = 4. Both are perfect squares.
-
Middle Term: 2ab = 2(3x)(4) = 24x. This matches the middle term of the trinomial.
-
Sign: The middle term is positive, so the factored form will use a plus sign.
-
Factored Form: (3x + 4)²
The Advantages of Using a Perfect Square Trinomial Calculator
While manual factoring builds understanding, a calculator offers significant advantages, particularly when dealing with:
-
Complex Trinomials: Calculations involving large numbers or fractions can become cumbersome manually. A calculator handles these complexities effortlessly.
-
Time Efficiency: A calculator provides instant results, saving valuable time, especially when working on multiple problems or under time constraints.
-
Accuracy: Manual calculations are prone to errors, especially with more intricate trinomials. A calculator minimizes the risk of human error, ensuring accurate results every time.
-
Learning Aid: A calculator can serve as a valuable learning tool. By comparing your manual calculations with the calculator's results, you can identify and correct errors in your understanding.
Choosing and Using a Perfect Square Trinomial Calculator
Several online calculators are readily available. When choosing a calculator, consider factors such as:
-
Ease of Use: The interface should be intuitive and straightforward. Complex interfaces can hinder efficient problem-solving.
-
Accuracy: Verify the calculator's accuracy by testing it with known examples.
-
Features: Some calculators offer additional features such as step-by-step solutions, which can further aid learning.
-
Accessibility: Ensure the calculator is accessible on various devices (desktops, tablets, smartphones).
To use the calculator, simply input the coefficients of the trinomial (a, b, and c in the standard form ax² + bx + c). The calculator will then output the factored form.
Beyond Basic Factoring: Extending Your Knowledge
Understanding perfect square trinomials forms the foundation for tackling more advanced algebraic concepts. Here are some areas to explore after mastering the basics:
-
Difference of Squares: This is another common type of factoring problem related to perfect squares. It involves factoring expressions in the form a² - b².
-
Completing the Square: This technique involves manipulating quadratic equations to create a perfect square trinomial, allowing you to solve for the roots of the equation.
-
Solving Quadratic Equations: Factoring, including factoring perfect square trinomials, is a fundamental method for solving quadratic equations.
-
Advanced Polynomial Factoring: Perfect square trinomial factoring is a stepping stone towards factoring more complex polynomials.
SEO Optimization and Keyword Integration
This guide is optimized for search engines using a range of relevant keywords and semantic variations. Key terms such as "perfect square trinomial calculator," "factoring perfect square trinomials," "algebra calculator," "quadratic equations," "polynomial factoring," and variations thereof are strategically incorporated throughout the text to improve search engine visibility.
Conclusion: Mastering Perfect Square Trinomials for Success in Algebra
Understanding and mastering perfect square trinomials is a critical skill in algebra. While manual factoring is important for conceptual understanding, utilizing a perfect square trinomial calculator enhances efficiency and accuracy. This combination of theoretical knowledge and practical application equips you to tackle more complex algebraic problems and achieve success in your mathematical endeavors. Remember to practice regularly and explore related topics to build a strong foundation in algebra. By combining manual calculation practice with the aid of a calculator, you can unlock a deeper understanding of this key algebraic concept. This approach maximizes efficiency and reinforces learning, allowing you to confidently tackle any perfect square trinomial problem you encounter.
Latest Posts
Latest Posts
-
What Is 77 Days From Today
Apr 06, 2025
-
What Percent Of 500 Is 20
Apr 06, 2025
-
What Is 240 Pounds In Kg
Apr 06, 2025
-
How Much Feet Is 57 Inches
Apr 06, 2025
-
Integral Of 1 1 U 2
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about Factoring A Perfect Square Trinomial Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
