Equations Of Parallel And Perpendicular Lines Calculator
Greels
Apr 01, 2025 · 6 min read
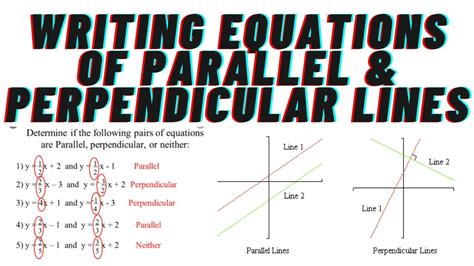
Table of Contents
Equations of Parallel and Perpendicular Lines Calculator: A Comprehensive Guide
Understanding parallel and perpendicular lines is fundamental in geometry and numerous applications across various fields, from architecture and engineering to computer graphics and data analysis. This article delves into the concepts of parallel and perpendicular lines, explaining how to find their equations manually and utilizing a hypothetical "Equations of Parallel and Perpendicular Lines Calculator" to streamline the process. We'll explore various scenarios and provide practical examples to solidify your understanding.
Understanding Parallel and Perpendicular Lines
Before diving into calculations, let's refresh our understanding of these crucial geometric concepts:
Parallel Lines: Two lines are parallel if they lie in the same plane and never intersect, no matter how far they are extended. A key characteristic is that they have the same slope.
Perpendicular Lines: Two lines are perpendicular if they intersect at a right angle (90 degrees). The slopes of perpendicular lines are negative reciprocals of each other. In other words, if the slope of one line is m, the slope of a line perpendicular to it is -1/m. A special case arises when one line is horizontal (slope = 0) and the other is vertical (undefined slope).
Finding Equations Manually: A Step-by-Step Guide
Let's review the process of manually calculating the equations of parallel and perpendicular lines. We'll use the slope-intercept form of a linear equation: y = mx + b, where m is the slope and b is the y-intercept.
1. Finding the Equation of a Parallel Line
To find the equation of a line parallel to a given line, we need:
- The equation of the given line: This allows us to determine its slope.
- A point on the parallel line: This point will be used to solve for the y-intercept.
Steps:
- Determine the slope (m) of the given line. If the equation is in slope-intercept form (y = mx + b), the slope is the coefficient of x. If the equation is in standard form (Ax + By = C), rearrange it to slope-intercept form to find the slope: m = -A/B.
- Since parallel lines have the same slope, the slope of the parallel line is also m.
- Substitute the coordinates (x₁, y₁) of the point on the parallel line and the slope (m) into the point-slope form: y - y₁ = m(x - x₁).
- Solve for y to obtain the equation of the parallel line in slope-intercept form (y = mx + b).
Example:
Find the equation of the line parallel to y = 2x + 3 that passes through the point (1, 5).
- The slope of the given line is m = 2.
- The slope of the parallel line is also m = 2.
- Using the point-slope form: y - 5 = 2(x - 1)
- Solving for y: y = 2x + 3
Notice that the equation of the parallel line is almost identical to the original line, differing only possibly in the y-intercept.
2. Finding the Equation of a Perpendicular Line
To find the equation of a line perpendicular to a given line, we also need:
- The equation of the given line: To determine its slope.
- A point on the perpendicular line: To solve for the y-intercept.
Steps:
- Determine the slope (m) of the given line. As above, convert to slope-intercept form if needed.
- Calculate the slope of the perpendicular line. This is the negative reciprocal of the given line's slope: m_perpendicular = -1/m.
- Substitute the coordinates (x₁, y₁) of the point on the perpendicular line and the perpendicular slope (m_perpendicular) into the point-slope form: y - y₁ = m_perpendicular(x - x₁).
- Solve for y to obtain the equation of the perpendicular line in slope-intercept form.
Example:
Find the equation of the line perpendicular to y = 2x + 3 that passes through the point (1, 5).
- The slope of the given line is m = 2.
- The slope of the perpendicular line is m_perpendicular = -1/2.
- Using the point-slope form: y - 5 = -1/2(x - 1)
- Solving for y: y = -1/2x + 5.5
The Hypothetical "Equations of Parallel and Perpendicular Lines Calculator"
While manual calculation is instructive, a calculator can significantly speed up the process, especially when dealing with complex equations or multiple calculations. Let's imagine a user-friendly "Equations of Parallel and Perpendicular Lines Calculator."
Features:
- Input: The calculator would accept the equation of the given line in various forms (slope-intercept, standard, or even point-slope). It would also require the coordinates of a point on the parallel or perpendicular line.
- Output: The calculator would provide the equations of both the parallel and perpendicular lines in slope-intercept form, along with a clear visual representation of the lines, potentially allowing users to adjust the viewing window and zoom levels.
- Error Handling: Robust error handling would address invalid inputs, such as non-linear equations or missing coordinates.
- Additional functionalities: This advanced calculator could calculate the angle between two lines, the distance between a point and a line, and the intersection point of two lines.
- User Interface: A clean and intuitive interface would be crucial for easy navigation and data input.
Workflow:
- Input the equation of the given line: The user would enter the equation using standard mathematical notation.
- Input the coordinates of the point: The user would input the x and y coordinates of the point through which the parallel and perpendicular lines will pass.
- Select the desired operation: The user would choose whether to calculate the equation of a parallel or perpendicular line.
- View the results: The calculator would instantly display the equations of the parallel and perpendicular lines, along with a visual graph.
Advanced Applications and Considerations
The ability to find parallel and perpendicular lines is crucial in numerous areas:
- Computer Graphics: Creating parallel and perpendicular lines is essential in generating 2D and 3D graphics.
- Engineering and Architecture: Calculating parallel and perpendicular lines plays a crucial role in structural design, ensuring stability and functionality.
- Data Analysis: In linear regression, the line of best fit can be used to find the equations of parallel lines for predicting outcomes based on various inputs.
- Game Development: Creating accurate representations of objects and their movements relies heavily on understanding and manipulating lines in 3D space.
Beyond the basic concepts, we can explore more complex scenarios such as dealing with lines in three-dimensional space, finding lines parallel or perpendicular to planes, or handling cases involving curves and other geometric shapes.
Conclusion
Understanding parallel and perpendicular lines is a foundational concept with far-reaching applications. While manual calculations provide valuable insights into the underlying mathematics, tools like the hypothetical "Equations of Parallel and Perpendicular Lines Calculator" streamline the process, enabling users to focus on the practical applications of this critical geometric principle. Mastering these concepts and utilizing available computational tools will empower you to tackle advanced problems across various disciplines. Remember to always check your work and utilize multiple approaches to ensure accuracy.
Latest Posts
Latest Posts
-
185 Pounds Is How Many Kg
Apr 02, 2025
-
100 Ft Equals How Many Meters
Apr 02, 2025
-
How Many Inches In 105 Cm
Apr 02, 2025
-
How Many Cm Is 76 Inches
Apr 02, 2025
-
How Many Inches In 71 Cm
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Equations Of Parallel And Perpendicular Lines Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
