Difference Quotient Calculator Step By Step
Greels
Apr 03, 2025 · 4 min read
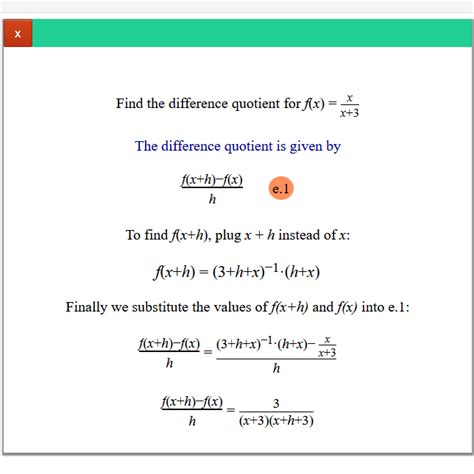
Table of Contents
Difference Quotient Calculator: A Step-by-Step Guide
The difference quotient is a fundamental concept in calculus, representing the average rate of change of a function over an interval. Understanding and calculating the difference quotient is crucial for grasping the core ideas of derivatives and limits. This comprehensive guide will walk you through the concept, provide a step-by-step approach to calculating it, and offer examples to solidify your understanding. We'll also explore different forms of the difference quotient and its applications.
What is the Difference Quotient?
The difference quotient measures the average rate of change of a function, f(x), over an interval. Imagine a curve representing your function. The difference quotient finds the slope of the secant line connecting two points on this curve. This slope approximates the instantaneous rate of change (the derivative) as the interval becomes infinitesimally small.
Mathematically, the difference quotient is defined as:
[f(x + h) - f(x)] / h
Where:
- f(x) is the function.
- x is a point on the function.
- h represents the change in x (the width of the interval). As h approaches 0, this approaches the instantaneous rate of change at x.
Step-by-Step Calculation of the Difference Quotient
Let's break down the calculation process with a detailed example. We'll use the function f(x) = x² + 2x - 3.
Step 1: Find f(x + h)
This involves substituting (x + h) into the function wherever you see x:
f(x + h) = (x + h)² + 2(x + h) - 3
Expanding this expression:
f(x + h) = x² + 2xh + h² + 2x + 2h - 3
Step 2: Find f(x + h) - f(x)
Subtract the original function, f(x), from the expanded f(x + h):
f(x + h) - f(x) = (x² + 2xh + h² + 2x + 2h - 3) - (x² + 2x - 3)
Simplify by canceling out like terms:
f(x + h) - f(x) = 2xh + h² + 2h
Step 3: Divide by h
Finally, divide the result from Step 2 by h:
[f(x + h) - f(x)] / h = (2xh + h² + 2h) / h
Simplify by canceling out h from each term (assuming h ≠ 0):
[f(x + h) - f(x)] / h = 2x + h + 2
Step 4: Analyze the Result
The simplified expression, 2x + h + 2, represents the difference quotient for the function f(x) = x² + 2x - 3. Notice that it still depends on h. As h approaches 0 (meaning we're considering a smaller and smaller interval), the difference quotient approaches 2x + 2. This limit, as h approaches 0, is the derivative of f(x), representing the instantaneous rate of change at any point x.
Examples with Different Functions
Let's explore a few more examples to solidify your understanding.
Example 1: Linear Function
Let f(x) = 3x + 5
- f(x + h) = 3(x + h) + 5 = 3x + 3h + 5
- f(x + h) - f(x) = (3x + 3h + 5) - (3x + 5) = 3h
- [f(x + h) - f(x)] / h = 3h / h = 3
The difference quotient for a linear function is simply its slope.
Example 2: Cubic Function
Let f(x) = x³ - 4x
- f(x + h) = (x + h)³ - 4(x + h) = x³ + 3x²h + 3xh² + h³ - 4x - 4h
- f(x + h) - f(x) = (x³ + 3x²h + 3xh² + h³ - 4x - 4h) - (x³ - 4x) = 3x²h + 3xh² + h³ - 4h
- [f(x + h) - f(x)] / h = (3x²h + 3xh² + h³ - 4h) / h = 3x² + 3xh + h² - 4
As h approaches 0, the difference quotient approaches 3x² - 4, which is the derivative of f(x) = x³ - 4x.
Example 3: Function with a Square Root
Let f(x) = √x
- f(x + h) = √(x + h)
- f(x + h) - f(x) = √(x + h) - √x
- [f(x + h) - f(x)] / h = (√(x + h) - √x) / h
This requires rationalizing the numerator:
Multiply the numerator and denominator by the conjugate of the numerator (√(x + h) + √x):
[(√(x + h) - √x)(√(x + h) + √x)] / [h(√(x + h) + √x)] = (x + h - x) / [h(√(x + h) + √x)] = h / [h(√(x + h) + √x)] = 1 / (√(x + h) + √x)
As h approaches 0, the difference quotient approaches 1 / (2√x).
Alternative Forms of the Difference Quotient
While the standard form is [f(x + h) - f(x)] / h, you might encounter alternative notations:
-
[f(x) - f(a)] / (x - a): This form focuses on the average rate of change between two specific points, a and x, on the function.
-
[f(a + h) - f(a)] / h: This is similar to the standard form but explicitly uses a specific point 'a' instead of a general 'x'.
Applications of the Difference Quotient
The difference quotient isn't just a theoretical concept; it has practical applications in various fields:
- Physics: Calculating average velocity and acceleration.
- Economics: Analyzing marginal cost and revenue.
- Engineering: Modeling rates of change in various systems.
- Computer Science: Numerical differentiation and approximation of derivatives.
Conclusion
The difference quotient is a cornerstone of calculus. Mastering its calculation is vital for understanding derivatives and their applications. By following the step-by-step approach outlined in this guide and practicing with various functions, you'll build a strong foundation in calculus and its practical uses. Remember that the limit of the difference quotient as h approaches zero gives you the derivative, a powerful tool for analyzing the instantaneous rate of change of a function. This understanding unlocks a deeper appreciation for the dynamics of change across numerous disciplines.
Latest Posts
Latest Posts
-
How Many Pounds Is 91 Kilos
Apr 04, 2025
-
How Many Feet Is 10000 M
Apr 04, 2025
-
How Many Lbs Is 2 3 Kg
Apr 04, 2025
-
How Many Square Feet Is 23 Acres
Apr 04, 2025
-
What Is 18 Inches In Mm
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Difference Quotient Calculator Step By Step . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
