Derivative Of X 2 4 2
Greels
Apr 06, 2025 · 4 min read
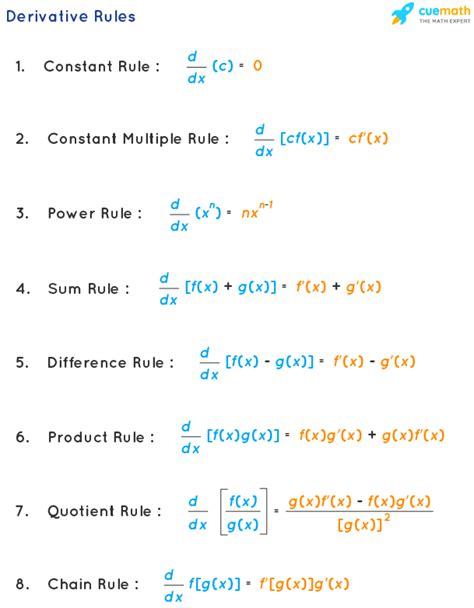
Table of Contents
Understanding the Derivative of x² + 4x + 2: A Comprehensive Guide
The derivative of a function describes its instantaneous rate of change at any given point. Understanding derivatives is fundamental to calculus and has wide-ranging applications in various fields, from physics and engineering to economics and finance. This article delves into the derivative of the function f(x) = x² + 4x + 2, explaining the process step-by-step and exploring its implications.
What is a Derivative?
Before diving into the specifics of our function, let's briefly review the concept of a derivative. The derivative of a function at a point represents the slope of the tangent line to the graph of the function at that point. Geometrically, it measures the instantaneous rate at which the function's value is changing. Analytically, it's defined as the limit of the difference quotient as the change in x approaches zero:
f'(x) = lim (Δx → 0) [(f(x + Δx) - f(x)) / Δx]
This limit, if it exists, is the derivative of f(x) with respect to x, often denoted as f'(x) or df/dx. The process of finding the derivative is called differentiation.
Differentiating Polynomials: The Power Rule
Our function, f(x) = x² + 4x + 2, is a polynomial. Polynomials are exceptionally easy to differentiate because of the power rule. The power rule states that the derivative of x<sup>n</sup> is nx<sup>n-1</sup>, where n is any real number.
Let's break down the differentiation process step-by-step:
Step 1: Differentiating x²
Applying the power rule, the derivative of x² is:
d(x²)/dx = 2x<sup>2-1</sup> = 2x
Step 2: Differentiating 4x
The term 4x can be written as 4x<sup>1</sup>. Applying the power rule:
d(4x)/dx = 4 * 1x<sup>1-1</sup> = 4x<sup>0</sup> = 4
Step 3: Differentiating the Constant Term 2
The derivative of a constant is always zero. This is because a constant has no change in value. Therefore:
d(2)/dx = 0
Step 4: Combining the Derivatives
To find the derivative of the entire function, we sum the derivatives of each term:
f'(x) = d(x² + 4x + 2)/dx = 2x + 4 + 0 = 2x + 4
Therefore, the derivative of f(x) = x² + 4x + 2 is f'(x) = 2x + 4.
Interpreting the Derivative: Slope and Rate of Change
The derivative, f'(x) = 2x + 4, provides valuable information about the original function, f(x) = x² + 4x + 2:
-
Slope of the Tangent Line: At any given point x, f'(x) represents the slope of the tangent line to the graph of f(x) at that point. For example, at x = 1, the slope of the tangent line is f'(1) = 2(1) + 4 = 6.
-
Rate of Change: The derivative also represents the instantaneous rate of change of f(x) with respect to x. This means it tells us how much f(x) changes for a small change in x at any particular point. A positive derivative indicates an increasing function, while a negative derivative indicates a decreasing function.
-
Critical Points: Setting the derivative equal to zero, f'(x) = 0, helps us find critical points of the function. These are points where the function may have a local maximum or minimum. In our case:
2x + 4 = 0 2x = -4 x = -2
At x = -2, the function has a critical point. Further analysis (using the second derivative test) would determine whether this is a local maximum or minimum.
Applications of the Derivative
The derivative of f(x) = x² + 4x + 2, and the concept of derivatives in general, have numerous applications:
-
Optimization Problems: In business and engineering, derivatives are used to find maximum or minimum values. For instance, finding the production level that maximizes profit or the dimensions of a container that minimize material usage.
-
Physics: Derivatives are crucial in physics, representing velocity (derivative of position) and acceleration (derivative of velocity).
-
Economics: In economics, derivatives are used to model marginal cost, marginal revenue, and other important economic concepts.
-
Machine Learning: Derivative-based optimization algorithms, such as gradient descent, are fundamental to training machine learning models.
Higher-Order Derivatives
We can also find higher-order derivatives of f(x). The second derivative, f''(x), is the derivative of the first derivative, f'(x). In our case:
f''(x) = d(2x + 4)/dx = 2
The second derivative represents the rate of change of the slope. A constant second derivative, as in this case, indicates a constant rate of change of the slope – meaning the function is a parabola.
Conclusion: The Significance of Understanding Derivatives
Understanding the derivative of x² + 4x + 2, and derivatives in general, is crucial for comprehending the behavior of functions. It provides powerful tools for analyzing rates of change, finding optimal values, and solving problems across diverse fields. The ability to differentiate simple polynomial functions, like this one, forms a solid foundation for tackling more complex differentiation problems and applying calculus to real-world scenarios. This foundational knowledge is essential for anyone pursuing studies or careers in fields that utilize mathematics and its applications. The seemingly simple function f(x) = x² + 4x + 2 thus serves as a gateway to a much broader and fascinating world of mathematical analysis and problem-solving. Mastering its derivative is a crucial stepping stone in this journey.
Latest Posts
Latest Posts
-
How Many Miles Is 50000 Km
Apr 06, 2025
-
3a 6a 2 8a 20 5a
Apr 06, 2025
-
52 Inches In Feet And Inches
Apr 06, 2025
-
How Many Hours Is 3600 Seconds
Apr 06, 2025
-
How Many Miles In 24 Km
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about Derivative Of X 2 4 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
