Derivative Of 1 + Sin X
Greels
Mar 30, 2025 · 5 min read
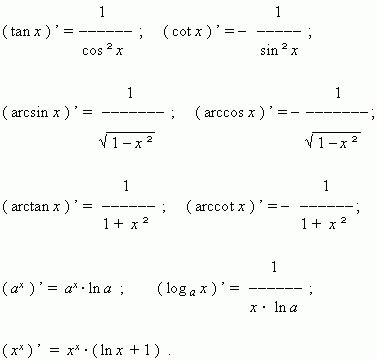
Table of Contents
Finding the Derivative of 1 + sin x: A Comprehensive Guide
The seemingly simple function, 1 + sin x, offers a rich opportunity to explore fundamental concepts in calculus, specifically differentiation. This comprehensive guide will delve into finding its derivative, explaining the underlying principles and providing numerous examples to solidify your understanding. We'll also touch upon applications and explore related concepts to build a strong foundation.
Understanding Derivatives: A Quick Refresher
Before diving into the specifics of 1 + sin x, let's briefly review the concept of a derivative. In simpler terms, the derivative of a function represents its instantaneous rate of change at any given point. Geometrically, it represents the slope of the tangent line to the function's graph at that point. The process of finding a derivative is called differentiation.
We use the notation f'(x) (pronounced "f prime of x") or dy/dx (pronounced "dy by dx") to denote the derivative of a function f(x) with respect to x.
Key Differentiation Rules: The Building Blocks
Several rules govern differentiation. Understanding these is crucial for tackling more complex functions. We'll focus on the rules relevant to our problem:
1. The Constant Rule:
The derivative of a constant is always zero. For example, if f(x) = 5, then f'(x) = 0. This makes intuitive sense: a constant function doesn't change, so its rate of change is zero.
2. The Sum/Difference Rule:
The derivative of a sum (or difference) of functions is the sum (or difference) of their derivatives. Mathematically:
If h(x) = f(x) + g(x), then h'(x) = f'(x) + g'(x).
Similarly, if h(x) = f(x) - g(x), then h'(x) = f'(x) - g'(x).
3. The Derivative of sin x:
This is the core piece of our puzzle. The derivative of sin x is cos x. This fundamental trigonometric derivative is derived using the limit definition of the derivative and trigonometric identities. Understanding its proof is beneficial, but for this article, we'll accept it as a given.
Deriving the Derivative of 1 + sin x
Now, armed with these rules, let's find the derivative of 1 + sin x. Let's define our function:
f(x) = 1 + sin x
Applying the sum rule, we differentiate each term separately:
The derivative of 1 (a constant) is 0.
The derivative of sin x is cos x.
Therefore:
f'(x) = 0 + cos x = cos x
Therefore, the derivative of 1 + sin x is cos x.
Illustrative Examples and Applications
Let's solidify our understanding with some examples and explore real-world applications:
Example 1: Finding the slope of the tangent line
Let's find the slope of the tangent line to the curve y = 1 + sin x at x = π/2.
Since f'(x) = cos x, we evaluate the derivative at x = π/2:
f'(π/2) = cos(π/2) = 0
The slope of the tangent line at x = π/2 is 0. This means the tangent line is horizontal at that point.
Example 2: Related Rates Problems
Imagine a lighthouse beam rotating at a constant rate. The distance the beam travels along a shore can be modeled using trigonometric functions. The rate of change of this distance can be found using the derivative of such a function, which might involve terms similar to 1 + sin x, if the model incorporates an offset or initial position.
Example 3: Analyzing Oscillatory Motion
Functions like 1 + sin x are frequently used to model simple harmonic motion (like a pendulum's swing). The derivative provides information about the velocity of the oscillating object at any given point in time.
Example 4: Signal Processing
In signal processing, the derivative of a signal (which might incorporate sinusoidal functions) is used to analyze its frequency components and identify changes in the signal over time.
Example 5: Economics and Finance
Economic models often use sinusoidal functions to represent cyclical trends. The derivative helps to understand the rate of change of these trends, such as the growth rate of a particular industry or the fluctuation in stock prices.
Exploring Further: Higher-Order Derivatives
While we've focused on the first derivative, it's possible to find higher-order derivatives. The second derivative, denoted f''(x) or d²y/dx², represents the rate of change of the first derivative. In our case:
f'(x) = cos x
f''(x) = -sin x
The third derivative would be:
f'''(x) = -cos x
And the fourth derivative would return us to the original function:
f''''(x) = sin x
This cyclical pattern of derivatives for trigonometric functions is a notable characteristic.
Understanding the Graph: Visualizing the Derivative
Plotting both the original function, f(x) = 1 + sin x, and its derivative, f'(x) = cos x, offers valuable insights. The graph of f(x) = 1 + sin x is a sine wave shifted vertically upwards by one unit. The graph of f'(x) = cos x is a cosine wave. Observe how the derivative's values correspond to the slope of the original function. Where the slope of f(x) is zero, the derivative f'(x) is also zero. Where f(x) is increasing, f'(x) is positive, and where f(x) is decreasing, f'(x) is negative.
Beyond the Basics: Expanding Our Understanding
This exploration of the derivative of 1 + sin x serves as a stepping stone to more complex differentiation problems. By mastering the fundamental rules and applying them strategically, you can successfully tackle a wider range of functions. Understanding the derivative's graphical representation and its real-world applications strengthens the understanding of calculus beyond mere symbolic manipulation.
This comprehensive guide has covered:
- The foundational concepts of derivatives.
- Essential differentiation rules (constant rule, sum rule, derivative of sin x).
- A step-by-step derivation of the derivative of 1 + sin x.
- Real-world applications and illustrative examples.
- An introduction to higher-order derivatives.
- A graphical interpretation of the derivative.
By mastering these concepts, you'll be well-equipped to handle increasingly complex differentiation problems in calculus and related fields. Remember that practice is key – work through numerous examples and challenges to solidify your understanding and build confidence in your analytical skills.
Latest Posts
Latest Posts
-
How Many Inches Is 23 Mm
Apr 01, 2025
-
What Day Is 150 Days From Today
Apr 01, 2025
-
Cuanto Es 76 Kilos En Libras
Apr 01, 2025
-
How Much Is 46 Kilos In Pounds
Apr 01, 2025
-
How Many Feet Are In 1500 Meters
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Derivative Of 1 + Sin X . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
