Adding / Subtracting Rational Expressions Calculator
Greels
Mar 26, 2025 · 5 min read
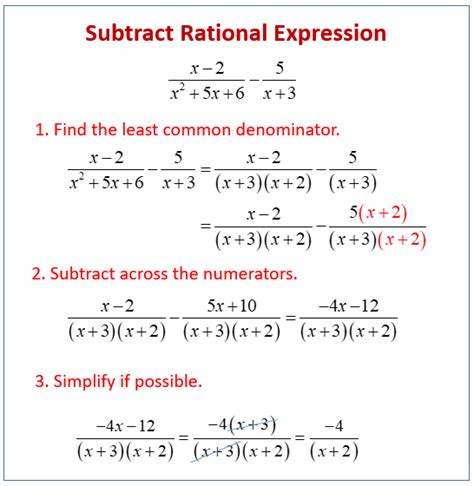
Table of Contents
Adding and Subtracting Rational Expressions Calculator: A Comprehensive Guide
Adding and subtracting rational expressions can be a daunting task for many students and even seasoned mathematicians. The process involves finding common denominators, simplifying expressions, and carefully managing signs. Fortunately, understanding the underlying principles and leveraging the power of online calculators can significantly simplify this process. This comprehensive guide will delve into the intricacies of adding and subtracting rational expressions, explain the step-by-step process, and demonstrate how to effectively use a rational expressions calculator.
Understanding Rational Expressions
Before diving into the intricacies of addition and subtraction, it's crucial to grasp the fundamental concept of rational expressions. A rational expression is simply a fraction where the numerator and the denominator are polynomials. For instance, (3x² + 2x + 1) / (x - 2) is a rational expression. Polynomials are expressions involving variables raised to non-negative integer powers, along with constants and coefficients.
Key Characteristics of Rational Expressions:
- Numerator: The polynomial on the top of the fraction.
- Denominator: The polynomial on the bottom of the fraction.
- Restrictions: The denominator cannot equal zero. Any values of the variable that would make the denominator zero are excluded from the domain of the rational expression. This is crucial to remember throughout all calculations.
Adding Rational Expressions: A Step-by-Step Approach
Adding rational expressions is analogous to adding regular fractions. The core principle remains the same: you need a common denominator before you can add the numerators.
Step 1: Find the Least Common Denominator (LCD)
The LCD is the smallest expression that contains all the factors of each denominator. This is akin to finding the least common multiple (LCM) in arithmetic. Let's illustrate with an example:
Add: (2x)/(x+3) + (x)/(x-1)
The denominators are (x+3) and (x-1). Since they share no common factors, the LCD is simply their product: (x+3)(x-1).
Step 2: Rewrite each fraction with the LCD
Now, rewrite each fraction with the LCD as the denominator. This involves multiplying both the numerator and denominator of each fraction by the appropriate factors.
(2x)/(x+3) * ((x-1)/(x-1)) + (x)/(x-1) * ((x+3)/(x+3))
This results in:
(2x(x-1))/((x+3)(x-1)) + (x(x+3))/((x+3)(x-1))
Step 3: Add the numerators
Now that both fractions have the same denominator, add the numerators:
(2x(x-1) + x(x+3))/((x+3)(x-1))
Step 4: Simplify the expression
Expand and simplify the numerator:
(2x² - 2x + x² + 3x)/((x+3)(x-1))
(3x² + x)/((x+3)(x-1))
This simplified expression is the sum of the original rational expressions. Always check for further simplification opportunities – you might be able to factor the numerator and cancel common factors with the denominator.
Subtracting Rational Expressions: A Similar Process
Subtracting rational expressions follows a very similar procedure. The key difference is that you're subtracting the numerators instead of adding them. Remember to be mindful of the signs, particularly when dealing with polynomials involving multiple terms.
Example:
Subtract: (3x)/(x+2) - (x-1)/(x-2)
Step 1: Find the LCD: (x+2)(x-2)
Step 2: Rewrite with the LCD:
(3x(x-2))/((x+2)(x-2)) - ((x-1)(x+2))/((x+2)(x-2))
Step 3: Subtract the numerators (be mindful of signs!):
(3x(x-2) - (x-1)(x+2))/((x+2)(x-2))
Step 4: Simplify:
(3x² - 6x - (x² + 2x - x - 2))/((x+2)(x-2))
(3x² - 6x - x² - x + 2)/((x+2)(x-2))
(2x² - 7x + 2)/((x+2)(x-2))
Utilizing a Rational Expressions Calculator
While understanding the manual process is crucial for building a solid mathematical foundation, utilizing an online rational expressions calculator can streamline the process, especially for complex expressions. These calculators are designed to handle intricate polynomials and provide accurate results.
Key Features to Look For in a Calculator:
- Input Flexibility: The calculator should accept various input formats, including both standard notation and potentially more advanced mathematical syntax.
- Step-by-Step Solutions: A good calculator will not just provide the final answer but also display the step-by-step process, allowing you to learn from the calculations. This is invaluable for understanding the underlying principles.
- Error Handling: The calculator should be robust enough to handle various input errors and provide informative messages to guide you in correcting any mistakes.
- Visualization: Some advanced calculators may even offer visual representations of the expressions, which can greatly improve comprehension.
Advanced Scenarios and Considerations
While the above examples demonstrate the fundamental principles, more complex scenarios may arise. Here are some considerations:
- Complex Fractions: Rational expressions can be nested within other rational expressions, creating complex fractions. In such cases, it’s often helpful to simplify the numerator and denominator separately before proceeding with addition or subtraction.
- Factoring Challenges: Sometimes factoring the polynomials in the numerator or denominator can be difficult. Mastering various factoring techniques, such as quadratic factoring, difference of squares, and grouping, is crucial.
- Partial Fraction Decomposition: For more advanced applications, you might encounter partial fraction decomposition, where a complex rational expression is broken down into simpler fractions. This is frequently used in calculus.
Practical Applications of Rational Expressions
Rational expressions are far from just abstract mathematical concepts. They find significant applications in various fields:
- Physics: Solving problems involving motion, electricity, and other physical phenomena often involves rational expressions.
- Engineering: Rational expressions are essential in designing and analyzing structures, circuits, and systems.
- Economics: Economic models frequently incorporate rational expressions to represent relationships between variables.
- Computer Science: Algorithms and data structures often involve rational expressions in their analysis.
Conclusion: Mastering Rational Expressions
Adding and subtracting rational expressions is a fundamental skill in algebra and beyond. While mastering the manual process is important for a deep understanding, utilizing online calculators can significantly enhance efficiency and accuracy, particularly when dealing with more complex problems. Remember to always check your work carefully and understand the underlying principles to build a strong mathematical foundation. By combining manual calculations with the assistance of technology, you can effectively tackle even the most challenging rational expression problems and apply this knowledge to various real-world applications.
Latest Posts
Latest Posts
-
How Far Is 80 Kilometers In Miles
Mar 29, 2025
-
How Many Ounces In 250 G
Mar 29, 2025
-
2 4 Km Is How Many Miles
Mar 29, 2025
-
200 M Is How Many Feet
Mar 29, 2025
-
How Many Ft Is 62 Inches
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about Adding / Subtracting Rational Expressions Calculator . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
