32 6 As A Mixed Number
Greels
Apr 23, 2025 · 4 min read
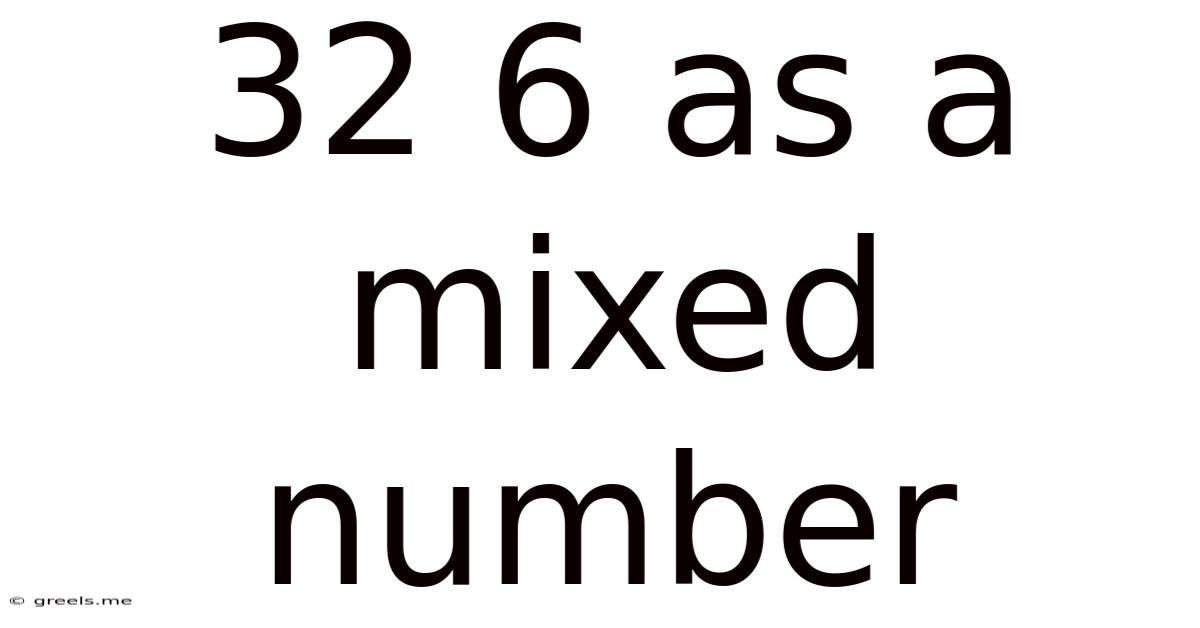
Table of Contents
32/6 as a Mixed Number: A Comprehensive Guide
Understanding fractions and how to convert them into mixed numbers is a fundamental skill in mathematics. This comprehensive guide will delve into the process of converting the improper fraction 32/6 into a mixed number, explaining the steps involved and providing various examples to solidify your understanding. We'll also explore the concept of simplifying fractions and its importance in mathematical operations.
What is a Mixed Number?
A mixed number combines a whole number and a proper fraction. A proper fraction is a fraction where the numerator (the top number) is smaller than the denominator (the bottom number). For example, 1 ½, 2 ¾, and 5 ⅓ are all mixed numbers. They represent a quantity greater than one.
What is an Improper Fraction?
An improper fraction is a fraction where the numerator is greater than or equal to the denominator. For example, 32/6 is an improper fraction because the numerator (32) is greater than the denominator (6). Improper fractions represent a quantity greater than or equal to one.
Converting 32/6 to a Mixed Number: Step-by-Step
Converting an improper fraction like 32/6 into a mixed number involves dividing the numerator by the denominator. Here's how:
Step 1: Divide the Numerator by the Denominator
Divide 32 by 6:
32 ÷ 6 = 5 with a remainder of 2
Step 2: Identify the Whole Number and the Remainder
- The whole number is the quotient (the result of the division) which is 5.
- The remainder is 2.
Step 3: Form the Mixed Number
The whole number (5) becomes the whole number part of the mixed number. The remainder (2) becomes the numerator of the proper fraction, and the denominator remains the same (6).
Therefore, 32/6 as a mixed number is 5 ⅔.
Visualizing the Conversion
Imagine you have 32 cookies, and you want to divide them equally among 6 friends. You can give each friend 5 cookies (5 x 6 = 30 cookies). You'll have 2 cookies left over (32 - 30 = 2). This leftover represents the fraction ⅔. So each friend gets 5 whole cookies and ⅔ of a cookie. This visually represents the mixed number 5 ⅔.
Simplifying Fractions: An Important Consideration
Before converting an improper fraction to a mixed number, it’s often beneficial to simplify the fraction first. Simplifying a fraction means reducing it to its lowest terms by finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it.
Let's look at 32/6. Both 32 and 6 are divisible by 2.
- 32 ÷ 2 = 16
- 6 ÷ 2 = 3
This simplifies the fraction to 16/3. Now, let's convert 16/3 to a mixed number:
16 ÷ 3 = 5 with a remainder of 1
Therefore, 16/3 as a mixed number is 5 ⅓. Notice that we arrive at the same result as before, but simplifying first often makes the division easier.
Practical Applications of Converting Improper Fractions to Mixed Numbers
Converting improper fractions to mixed numbers is crucial in various real-life scenarios and mathematical problems:
-
Measurement: Imagine you're measuring a length of 32/6 meters. Expressing this as 5 ⅔ meters is more intuitive and easier to understand.
-
Baking: A recipe might call for 32/6 cups of flour. Converting this to 5 ⅔ cups makes measuring significantly easier.
-
Sharing: Distributing items equally among individuals frequently involves improper fractions. Converting these to mixed numbers helps visualize the distribution more clearly.
Further Examples
Let's practice with a few more examples:
-
45/8: 45 ÷ 8 = 5 with a remainder of 5. Therefore, 45/8 = 5 ⅝.
-
27/4: 27 ÷ 4 = 6 with a remainder of 3. Therefore, 27/4 = 6 ¾.
-
61/10: 61 ÷ 10 = 6 with a remainder of 1. Therefore, 61/10 = 6 ⅒.
-
100/7: 100 ÷ 7 = 14 with a remainder of 2. Therefore, 100/7 = 14 ⅔.
Converting Mixed Numbers Back to Improper Fractions
It's also important to understand how to convert a mixed number back into an improper fraction. This involves the following steps:
-
Multiply the whole number by the denominator: For example, in 5 ⅔, multiply 5 x 6 = 30.
-
Add the numerator: Add the result from step 1 to the numerator: 30 + 2 = 32.
-
Keep the denominator the same: The denominator remains 6.
Therefore, 5 ⅔ becomes 32/6.
Troubleshooting Common Mistakes
-
Incorrect division: Double-check your division to ensure accuracy. A small error in division will lead to an incorrect mixed number.
-
Forgetting the remainder: Remember to include the remainder as the numerator of the proper fraction.
-
Incorrect simplification: Always simplify the fraction before converting to a mixed number to make the calculation easier and the result clearer.
Conclusion
Converting improper fractions to mixed numbers is a valuable skill in mathematics with wide-ranging applications. By following the steps outlined in this guide and practicing with various examples, you'll master this concept and be able to confidently tackle any improper fraction conversion. Remember the importance of simplifying fractions before conversion for easier calculations and a more concise final answer. Understanding both improper fractions and mixed numbers, and the ability to convert between them, is a crucial building block for more advanced mathematical concepts.
Latest Posts
Related Post
Thank you for visiting our website which covers about 32 6 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.