2 Step Equations Calculator With Fractions
Greels
Mar 27, 2025 · 5 min read
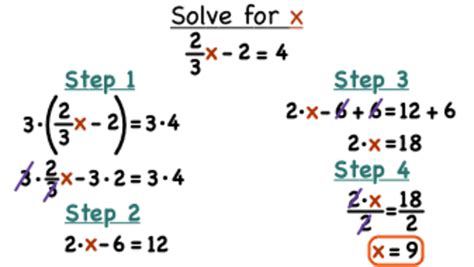
Table of Contents
Solving Two-Step Equations with Fractions: A Comprehensive Guide
Solving equations is a fundamental skill in algebra. While straightforward with whole numbers, incorporating fractions adds a layer of complexity. This comprehensive guide will walk you through the process of solving two-step equations containing fractions, providing clear explanations, practical examples, and valuable tips to enhance your understanding and problem-solving skills. We'll also explore the use of a two-step equation calculator with fractions as a tool to verify your solutions and improve your learning experience.
Understanding Two-Step Equations
A two-step equation is an algebraic equation that requires two steps to isolate the variable and solve for its value. The general form is: ax + b = c, where 'a', 'b', and 'c' are constants, and 'x' is the variable. When fractions are involved, the equation might look like: (2/3)x + 1/2 = 5/6.
Step-by-Step Solution Process
Let's break down the process of solving two-step equations with fractions using a systematic approach. We will use the example equation: (2/3)x + 1/2 = 5/6
Step 1: Isolate the Term with the Variable
Our goal is to get the term containing 'x' by itself on one side of the equation. In our example, this means eliminating the constant term, 1/2. To do this, we perform the inverse operation: subtraction. We subtract 1/2 from both sides of the equation:
(2/3)x + 1/2 - 1/2 = 5/6 - 1/2
This simplifies to:
(2/3)x = 5/6 - 1/2
Now we need to find a common denominator to subtract the fractions on the right-hand side. The common denominator for 6 and 2 is 6:
(2/3)x = 5/6 - 3/6
(2/3)x = 2/6
Further simplification gives:
(2/3)x = 1/3
Step 2: Solve for the Variable
Now that the term with 'x' is isolated, we need to solve for 'x'. In our example, 'x' is multiplied by 2/3. The inverse operation of multiplication is division. To isolate 'x', we divide both sides of the equation by 2/3:
x = (1/3) / (2/3)
Remember that dividing by a fraction is the same as multiplying by its reciprocal:
x = (1/3) * (3/2)
This simplifies to:
x = 3/6
And finally, simplifying the fraction:
x = 1/2
Therefore, the solution to the equation (2/3)x + 1/2 = 5/6 is x = 1/2.
Handling Different Fraction Scenarios
While the above example provides a clear path, let's explore scenarios that might present slightly different challenges.
Scenario 1: Negative Fractions
Equations can involve negative fractions. For example: -(1/4)x + 2/5 = 1.
The solution steps remain the same, but careful attention must be paid to the signs:
- Isolate the term with x: Subtract 2/5 from both sides:
-(1/4)x = 1 - 2/5 = 3/5 - Solve for x: Divide both sides by -(1/4) (or multiply by -4):
x = (3/5) / (-1/4) = (3/5) * (-4) = -12/5
Scenario 2: Fractions with Variables in the Denominator
Equations like 5/(x-2) = 10 require a different approach. Here, we start by eliminating the denominator by multiplying both sides by (x-2):
5 = 10(x-2)
5 = 10x - 20
Then solve for x using standard two-step equation methods.
Scenario 3: Mixed Numbers
Mixed numbers should be converted to improper fractions before solving the equation. For example, convert 1 1/2 to 3/2 before applying the solving steps.
Using a Two-Step Equation Calculator with Fractions
While mastering the manual process is crucial, a two-step equation calculator with fractions can serve as a valuable tool. These calculators can verify your solutions, provide step-by-step demonstrations, and help you understand the process visually. However, relying solely on calculators without understanding the underlying concepts is not advisable. Use the calculator to confirm your work and identify potential errors, not as a replacement for learning the techniques.
Advanced Techniques and Tips
Let's explore some strategies to streamline the process and handle more complex equations.
Clearing Fractions: The LCM Method
To avoid working with fractions throughout the entire process, you can clear the fractions at the beginning using the Least Common Multiple (LCM) of the denominators. In the equation (2/3)x + 1/2 = 5/6, the LCM of 3, 2, and 6 is 6. Multiply each term by 6:
6 * (2/3)x + 6 * (1/2) = 6 * (5/6)
This simplifies to:
4x + 3 = 5
Now the equation is free of fractions, making the solution easier.
Checking Your Work
Always check your solution by substituting the value back into the original equation. If the equation holds true, your solution is correct. For example, in our original equation, substitute x = 1/2:
(2/3)*(1/2) + 1/2 = 1/3 + 1/2 = (2+3)/6 = 5/6
This confirms that x = 1/2 is the correct solution.
Common Mistakes to Avoid
Several common errors can hinder your ability to solve these equations correctly. Here are some pitfalls to watch out for:
- Incorrectly applying the order of operations (PEMDAS/BODMAS): Remember to follow the order of operations correctly.
- Making mistakes with signs: Pay close attention to negative signs when adding, subtracting, multiplying, and dividing fractions.
- Incorrectly simplifying fractions: Always reduce fractions to their simplest form.
- Not checking your solution: Always substitute your solution back into the original equation to verify its accuracy.
Conclusion
Solving two-step equations with fractions requires a systematic approach, a solid understanding of fraction operations, and attention to detail. While a two-step equation calculator with fractions can be a valuable aid, mastering the manual process is key to developing your algebraic skills. By practicing regularly and utilizing the strategies and techniques discussed in this guide, you will build confidence and proficiency in handling these types of equations. Remember to always check your work and learn from any mistakes you make. The journey to mastering algebra is one of continuous learning and practice.
Latest Posts
Latest Posts
-
What Is 133 Kg In Pounds
Mar 30, 2025
-
How Many Inches In 55 Cm
Mar 30, 2025
-
2 4w 1 10 W 3 4
Mar 30, 2025
-
38 Pounds Is How Many Kilograms
Mar 30, 2025
-
177 Cm In Feet And Inches
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about 2 Step Equations Calculator With Fractions . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
