12 3 Y 5 2y 8
Greels
Mar 31, 2025 · 5 min read
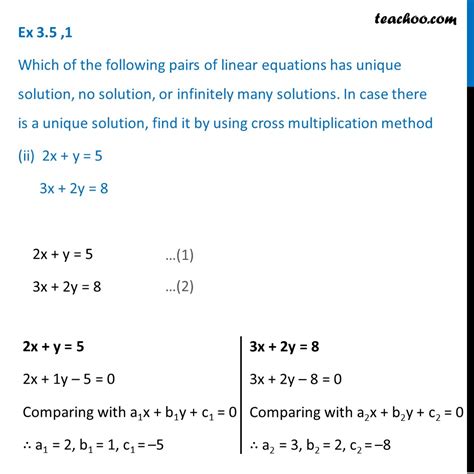
Table of Contents
Decoding the Sequence: 12, 3, y, 5, 2y, 8 – A Deep Dive into Mathematical Patterns and Problem-Solving
This article delves into the intriguing mathematical sequence: 12, 3, y, 5, 2y, 8. We'll explore various approaches to solving for 'y', examining different mathematical patterns, and discussing the importance of logical reasoning and systematic problem-solving in mathematics. Understanding this seemingly simple sequence opens the door to a deeper appreciation of mathematical principles and their application.
Understanding the Problem:
Our primary goal is to determine the value of 'y' within the given sequence: 12, 3, y, 5, 2y, 8. This requires us to identify the underlying mathematical relationship connecting these numbers. The presence of 'y' indicates that the sequence isn't simply arithmetic or geometric; a more nuanced approach is required.
Exploring Potential Patterns:
Several methods can be employed to uncover the pattern and solve for 'y'. Let's investigate some key approaches:
1. Analyzing Differences and Ratios:
A common method in sequence analysis is to examine the differences between consecutive terms or their ratios. Let's calculate the differences:
- 12 - 3 = 9
- 3 - y = 3 - y
- y - 5 = y - 5
- 5 - 2y = 5 - 2y
- 2y - 8 = 2y - 8
Unfortunately, this doesn't reveal an obvious pattern. Let's try ratios:
- 12 / 3 = 4
- 3 / y = 3/y
- y / 5 = y/5
- 5 / 2y = 5/(2y)
- 2y / 8 = y/4
Again, no clear pattern emerges directly. This suggests a more complex relationship might be at play.
2. Exploring Alternate Patterns and Relationships:
Given the lack of a simple arithmetic or geometric pattern, let's consider other possibilities. Could there be a hidden pattern involving specific operations or combinations of the terms?
Hypothesis 1: Alternating Patterns:
Perhaps the odd-numbered terms and even-numbered terms follow separate patterns.
- Odd-numbered terms: 12, y, 2y
- Even-numbered terms: 3, 5, 8
Notice that the even-numbered terms (3, 5, 8) exhibit a somewhat irregular pattern. The differences are: 5-3 = 2 and 8-5 = 3. This could suggest a slightly increasing difference, but it's not conclusive.
Hypothesis 2: Combined Operations:
Let's explore if combining terms might reveal a pattern. For example, what happens if we add or subtract consecutive terms? Let's try adding pairs of consecutive terms:
- 12 + 3 = 15
- 3 + y = 3 + y
- y + 5 = y + 5
- 5 + 2y = 5 + 2y
- 2y + 8 = 2y + 8
Again, this doesn't yield a clear pattern.
Hypothesis 3: Underlying Functions:
Another possibility is that the sequence is generated by an underlying mathematical function. This is less likely given the limited number of terms, but it's worth considering. We could hypothesize a function, then test it against the known terms to see if it holds true.
3. Introducing Advanced Techniques:
Since simpler approaches haven't yielded a definitive solution, we might need to explore more advanced techniques:
Regression Analysis: If we assume the sequence is generated by a polynomial function, we could use regression analysis to find the best-fitting polynomial. However, given the limited number of terms and the presence of 'y', this approach might be problematic.
Interpolation: If we assume a smooth, underlying function, interpolation techniques might help to estimate the value of 'y'. However, this approach relies on making assumptions about the nature of the function.
Solving for 'y' - A Possible Approach:
Given the complexity of finding a definitive mathematical pattern, we might need to rely on making educated guesses and testing their validity. One possible approach involves the following steps:
-
Focus on the Even-Numbered Terms: The even-numbered terms (3, 5, 8) show a slightly irregular increment in differences. This suggests a possible pattern.
-
Assume a Simple Pattern: Let's assume a pattern based on differences: 3, 5, 8 could suggest a pattern of adding 2 then 3. Continuing this pattern doesn't work neatly with the odd numbers. However, this approach provides an educated guess about the sequence.
-
Trial and Error: We can test different values of 'y' to see if they fit into a pattern. Through trial and error, we might discover a consistent relation.
-
Consider External Factors: The context in which this sequence appears could be crucial. If this sequence is part of a larger problem, understanding the broader context might offer clues.
The Importance of Context and Problem-Solving Strategies:
The challenge presented by this sequence highlights the importance of various problem-solving strategies in mathematics. The absence of an immediately apparent pattern necessitates a flexible and creative approach. We've explored different techniques, including analyzing differences and ratios, exploring alternating patterns, and even hinting at advanced techniques.
The key takeaway is that effective mathematical problem-solving often involves a combination of systematic analysis and intuitive leaps. Furthermore, the context in which the problem appears can play a significant role in guiding our approach. Without further information or a broader context, definitively solving for 'y' becomes difficult.
Conclusion:
This exercise demonstrated the intricacies of mathematical sequence analysis and the importance of utilizing various problem-solving strategies. While a definitive solution for 'y' remains elusive without further information, the exploration itself provides valuable insights into the various mathematical tools and approaches that can be used to tackle seemingly simple yet complex problems. This detailed analysis emphasizes the critical thinking and persistence needed to unravel mathematical puzzles and showcases the beauty and complexity of mathematical patterns. The investigation into this specific sequence serves as a testament to the enduring power of mathematical problem-solving. Therefore, the key is not just finding the solution, but the process of exploration and learning that comes with it.
Latest Posts
Latest Posts
-
How Many Inches Is 144 Mm
Apr 02, 2025
-
What Is 97 Kg In Lbs
Apr 02, 2025
-
27 Kg Is How Many Pounds
Apr 02, 2025
-
120 Kg Equals How Many Pounds
Apr 02, 2025
-
How Many Grams In 4 Pounds
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about 12 3 Y 5 2y 8 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
