10 7x - X - 7
Greels
Apr 03, 2025 · 5 min read
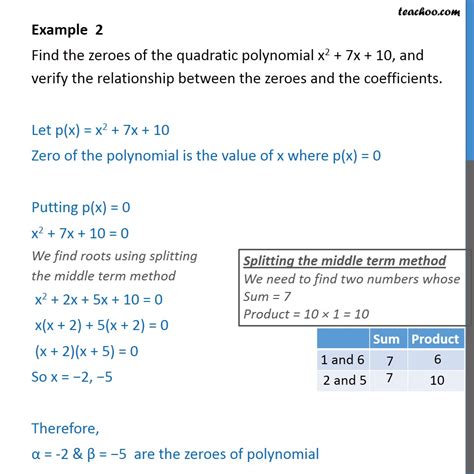
Table of Contents
Delving Deep into the Quadratic Equation: 107x - x - 7 = 0
The seemingly simple quadratic equation, 107x - x - 7 = 0, presents a fascinating opportunity to explore various mathematical concepts and techniques. While the equation itself might appear straightforward, its solution and the underlying principles involved offer a rich learning experience. This article will delve into a comprehensive analysis of this equation, covering simplification, solution methods, graphical representation, and the broader implications within the field of algebra.
Simplifying the Equation
Before embarking on the solution process, simplifying the equation is crucial. The equation, as presented, contains like terms that can be combined. We have 107x and -x. Combining these terms, we obtain:
106x - 7 = 0
This simplified form significantly improves the clarity and ease of solving the equation. This step exemplifies an important principle in mathematics: always simplify before proceeding to more complex steps. This simplification reduces computational errors and enhances understanding.
Solving the Equation: Methods and Approaches
Several methods can be employed to solve the simplified quadratic equation, 106x - 7 = 0. Let's explore two common approaches:
1. Isolation Method
The most straightforward approach is isolating the variable 'x'. This involves manipulating the equation algebraically to solve for x.
-
Add 7 to both sides: This eliminates the constant term on the left-hand side, leaving us with 106x = 7.
-
Divide both sides by 106: This isolates 'x', giving us the solution:
x = 7/106
This fractional representation is the exact solution. For practical applications, this fraction can be converted to a decimal approximation using a calculator:
x ≈ 0.0660377
This method is particularly efficient for linear equations, where the highest power of the variable is 1. Its simplicity and directness make it a preferred method for such equations.
2. Quadratic Formula (Though Not Strictly Necessary)
While the equation is linear after simplification, it's instructive to consider how the quadratic formula would be applied if we were to treat it as a quadratic equation in standard form (ax² + bx + c = 0). Our simplified equation can be written as:
0x² + 106x - 7 = 0
Here, a = 0, b = 106, and c = -7. The quadratic formula is:
x = (-b ± √(b² - 4ac)) / 2a
Plugging in the values, we get:
x = (-106 ± √(106² - 4 * 0 * -7)) / (2 * 0)
Notice the denominator becomes zero, indicating that the quadratic formula is not directly applicable to this simplified linear equation. This highlights the importance of simplification before applying specific solution methods. Attempting to use the quadratic formula on an already simplified linear equation would be an inefficient and potentially misleading approach.
Graphical Representation and Interpretation
The equation 106x - 7 = 0 can also be represented graphically. This provides a visual understanding of the solution. The equation represents a straight line. Plotting this line on a Cartesian coordinate system reveals the x-intercept, which corresponds to the solution of the equation.
The x-intercept is the point where the line crosses the x-axis (where y = 0). Since the equation is 106x - 7 = 0, the x-intercept occurs at x = 7/106. This visual representation reinforces the solution obtained algebraically. The graph clearly shows the single point where the line intersects the x-axis, confirming the unique solution to the equation.
Broader Mathematical Concepts
Solving this equation, even in its simplified form, touches upon several important mathematical concepts:
-
Linear Equations: The equation, after simplification, is a linear equation, characterized by its first-degree polynomial. Understanding linear equations is fundamental to algebra and numerous applications in science and engineering.
-
Equivalence Transformations: The process of adding 7 to both sides and dividing by 106 exemplifies equivalence transformations. These transformations maintain the equality of both sides of the equation, allowing us to manipulate the equation while preserving its solution.
-
Roots and Solutions: The value of x that satisfies the equation is referred to as its root or solution. The concept of roots is central to solving various types of equations, including quadratic, cubic, and higher-degree polynomials.
-
Algebraic Manipulation: Successfully solving the equation requires proficiency in basic algebraic manipulations, such as adding, subtracting, multiplying, and dividing both sides of an equation. These skills form the foundation of algebraic problem-solving.
Practical Applications
Linear equations like this one have a wide range of applications in various fields:
-
Physics: Describing motion, calculating forces, and analyzing circuits.
-
Engineering: Designing structures, modeling systems, and solving optimization problems.
-
Economics: Analyzing market trends, forecasting demand, and managing resources.
-
Computer Science: Developing algorithms, solving optimization problems, and creating simulations.
The ability to confidently solve such equations is therefore a crucial skill for students and professionals in numerous disciplines.
Extending the Concept: Exploring Variations
Let's consider variations of the original equation to further explore related concepts.
What if the equation was 107x² - x - 7 = 0? This would be a true quadratic equation, requiring the use of the quadratic formula or factoring techniques to find its solutions. The presence of the x² term introduces the possibility of two distinct real roots, one real root (repeated), or even complex roots, depending on the discriminant (b² - 4ac).
What if the equation included additional terms, like 107x - x - 7 + 5 = 0? This would again require simplification before applying appropriate solution methods. The process of simplification remains crucial to solving equations efficiently and accurately.
Conclusion: A Simple Equation, Profound Lessons
The simple equation 107x - x - 7 = 0, while appearing elementary at first glance, serves as a powerful tool for illustrating fundamental algebraic principles and techniques. The process of simplifying, solving, and interpreting the solution graphically demonstrates essential concepts in algebra. Moreover, understanding the underlying mathematical concepts related to this seemingly simple equation builds a solid foundation for tackling more complex mathematical problems. The applications of such linear equations extend far beyond the classroom, highlighting the practical significance of mastering this foundational area of mathematics. The exploration of variations of this equation emphasizes the iterative nature of problem-solving and the adaptability of various mathematical techniques to different scenarios. Ultimately, the journey through the solution of this equation provides a valuable and enriching experience in mathematical understanding.
Latest Posts
Latest Posts
-
18x 2 3x 1 5x 16
Apr 04, 2025
-
How Many Inches Is 127 Cm
Apr 04, 2025
-
What Day Will It Be In 37 Days
Apr 04, 2025
-
62 Inches Is How Many Centimeters
Apr 04, 2025
-
1600 Meters Is How Many Feet
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about 10 7x - X - 7 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
